本條目中,向量 與純量 分別用粗體 與斜體 顯示。例如,位置向量通常用
r
{\displaystyle \mathbf {r} \,\!}
r
{\displaystyle r\,\!}
在古典力學 裏,拉普拉斯-龍格-冷次向量 (Laplace–Runge–Lenz vector;簡稱為LRL向量 )主要是用來描述,當一個物體環繞著另外一個物體運動時,軌道 的形狀與取向 。典型的例子是行星的環繞著太陽公轉 。在一個物理系統裏,假若兩個物體以萬有引力 交互作用,則LRL向量必定是一個運動常數 ,不管在軌道的任何位置,計算出來的LRL向量都一樣[ 1] 保守量 。更廣義地,在克卜勒問題 裏,由於兩個物體以連心力 交互作用,而連心力遵守平方反比定律 ,所以,LRL向量是一個保守量[ 2]
氫原子 是由兩個帶電粒子構成的。這兩個帶電粒子以遵守庫侖定律 的靜電力 互相作用.靜電力是一個標準的平方反比 連心力 。所以,氫原子 內部的微觀運動是一個克卜勒問題。在量子力學 的發展初期,薛丁格 還在思索他的薛丁格方程式 的時候,沃夫岡·包立 使用LRL向量,關鍵性地推導出氫原子的發射光譜 [ 3]
在古典力學 與量子力學 裏,因為物理系統的某一種對稱性 ,會產生 一個或多個對應的保守值。LRL向量也不例外。可是,它相對應的對稱性很特別;在數學裏,克卜勒問題等價於一個粒子自由地移動於四維空間的三維球面 [ 4] [ 5]
拉普拉斯-龍格-冷次向量是因皮埃爾-西蒙·拉普拉斯 、卡爾·龍格 與威廉·冷次 而命名。它又稱為拉普拉斯向量 ,龍格-冷次向量 ,或冷次向量 。有趣的是,LRL向量並不是這三位先生發現的!這向量曾經被重複地發現過好幾次[ 6] 天體力學 中無因次 的離心率向量 [ 7] 狹義相對論 ,或電磁場 ,甚至於不同類型的連心力 。
在一個物理系統裏,在任意保守 的連心力 的作用下(參閱保守力 ),一個粒子的運動,都會擁有至少四個運動常數 ;能量 與角動量
L
{\displaystyle \mathbf {L} }
分量 皆為運動常數。粒子的軌道被限制於一個平面。粒子的動量
p
{\displaystyle \mathbf {p} }
力中心點 的位置到粒子位置的位移
r
{\displaystyle \mathbf {r} }
L
{\displaystyle \mathbf {L} }
r
⋅
L
=
0
{\displaystyle \mathbf {r} \cdot \mathbf {L} =0}
LRL向量
A
{\displaystyle \mathbf {A} }
平方反比定律 時,
A
{\displaystyle \mathbf {A} }
[ 1]
A
{\displaystyle \mathbf {A} }
平方反比定律 ,則
A
{\displaystyle \mathbf {A} }
定義 一個廣義LRL向量,但是,這廣義向量通常並沒有解析解 ,假若有,也會是一個非常複雜的函數[ 8] [ 9]
在重要的克卜勒問題中,LRL向量
A
{\displaystyle \mathbf {A} }
天文軌道 ,例如行星的運動。然而,物理學家對它並不熟悉,這很可能是因為與動量與角動量相比,它的物理內涵比較難以被直覺地理解。因此,在過去三個世紀裏,它曾被重複地發現過許多次[ 6] 雅各布·赫爾曼 最先發表了關於LRL向量的論文。在推導一個軌道方程式的過程中,他計算出LRL向量的大小,
A
{\displaystyle A}
[ 10] 橢圓 軌道離心率 的關係。稍後,赫爾曼把這結果告訴約翰·白努利 ,他的恩師。白努利又更進一步地推導出LRL向量的方向。這樣,LRL向量得到了它的現代形式[ 11]
在那個世紀末尾,皮埃爾-西蒙·拉普拉斯 又重新地發現了LRL向量的保守性;稍微不同地,他的導引使用的是分析方法,而不是幾何方法[ 12] 威廉·哈密頓 推導出全等的離心率向量 [ 7] 速端曲線 顯示出,粒子動量向量的頭部呈圓形移動[ 13] 約西亞·吉布斯 ,應用向量分析 ,推導出同樣的向量[ 14] 卡爾·龍格 將吉布斯的導引,納入自己所寫的一本廣受歡迎的,關於向量的,德文教科書內,成為其中的一個例題[ 15] 威廉·冷次 發表了一篇關於氫原子 的舊量子論 的論文。在這篇論文中,他引用龍格所寫的教科書的例題為參考[ 16] 沃爾夫岡·包立 用LRL向量與矩陣力學 ,而不是薛丁格方程式 ,來推導氫 原子 的光譜 [ 3]
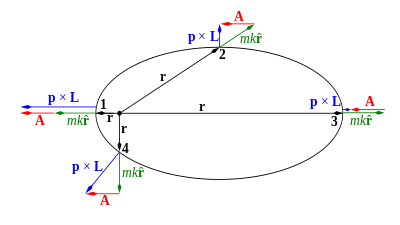
圖1:在平方反比連心力 的作用下,一個移動中的粒子,在橢圓軌道的四點(標記為1, 2, 3,與4)的LRL向量
A
{\displaystyle \mathbf {A} }
力中心點 表示為一個小黑點;從這黑點,位置向量
r
{\displaystyle \mathbf {r} }
角動量
L
{\displaystyle \mathbf {L} }
p
×
L
{\displaystyle \mathbf {p} \times \mathbf {L} }
m
k
r
^
{\displaystyle mk{\hat {\mathbf {r} }}}
A
{\displaystyle \mathbf {A} }
平方反比 連心力
F
(
r
)
{\displaystyle \mathbf {F} (r)}
F
(
r
)
=
−
k
r
2
r
^
{\displaystyle \mathbf {F} (r)=-{\frac {k}{r^{2}}}\mathbf {\hat {r}} }
其中,
k
{\displaystyle k}
r
^
=
r
r
{\displaystyle \mathbf {\hat {r}} ={\frac {\mathbf {r} }{r}}}
單位向量 ,
r
{\displaystyle \mathbf {r} }
位置向量 ,
r
{\displaystyle r}
r
{\displaystyle \mathbf {r} }
感受到此力的作用,一個粒子的軌道運動,其LRL向量的數學定義方程式為[ 1]
A
=
p
×
L
−
m
k
r
^
{\displaystyle \mathbf {A} =\mathbf {p} \times \mathbf {L} -mk\mathbf {\hat {r}} }
其中,
m
{\displaystyle m}
質量 ,
p
{\displaystyle \mathbf {p} }
動量 ,
L
=
r
×
p
{\displaystyle \mathbf {L} =\mathbf {r} \times \mathbf {p} }
角動量 。
由於平方反比連心力為保守力 ,能量
E
=
p
2
2
m
−
k
r
{\displaystyle E={\frac {p^{2}}{2m}}-{\frac {k}{r}}}
運動常數 :
d
E
d
t
=
p
m
p
˙
+
k
r
2
r
˙
=
0
{\displaystyle {\frac {\mathrm {d} E}{\mathrm {d} t}}={\frac {p}{m}}{\dot {p}}+{\frac {k}{r^{2}}}{\dot {r}}=0}
再者,角動量
L
{\displaystyle \mathbf {L} }
p
×
L
{\displaystyle \mathbf {p} \times \mathbf {L} }
r
{\displaystyle \mathbf {r} }
L
{\displaystyle \mathbf {L} }
A
{\displaystyle \mathbf {A} }
A
{\displaystyle \mathbf {A} }
這個單獨粒子的LRL向量定義,也可以延伸至像克卜勒問題一類的二體問題,只需要設定質量
m
{\displaystyle m}
約化質量 ,設定位置向量
r
{\displaystyle \mathbf {r} }
同樣的運動常數可以有很多種不同的表述.最常見的一種牽涉到離心率向量 。定義離心率向量
e
{\displaystyle \mathbf {e} }
m
k
{\displaystyle mk}
[ 7] [ 17]
e
=
A
m
k
=
1
m
k
(
p
×
L
)
−
r
^
{\displaystyle \mathbf {e} ={\frac {\mathbf {A} }{mk}}={\frac {1}{mk}}(\mathbf {p} \times \mathbf {L} )-\mathbf {\hat {r}} }
圖2:這是圖1的簡化版,角
θ
{\displaystyle \theta }
A
{\displaystyle \mathbf {A} }
r
{\displaystyle \mathbf {r} }
克卜勒問題的運動軌道,其形狀與取向 ,可以用LRL向量決定[ 1]
A
{\displaystyle \mathbf {A} }
r
{\displaystyle \mathbf {r} }
A
⋅
r
=
A
r
cos
θ
=
r
⋅
(
p
×
L
)
−
m
k
r
{\displaystyle \mathbf {A} \cdot \mathbf {r} =Ar\cos \theta =\mathbf {r} \cdot \left(\mathbf {p} \times \mathbf {L} \right)-mkr}
其中,
θ
{\displaystyle \theta }
A
{\displaystyle \mathbf {A} }
r
{\displaystyle \mathbf {r} }
置換 其三重積 ,
r
⋅
(
p
×
L
)
=
L
⋅
(
r
×
p
)
=
L
⋅
L
=
L
2
{\displaystyle \mathbf {r} \cdot \left(\mathbf {p} \times \mathbf {L} \right)=\mathbf {L} \cdot \left(\mathbf {r} \times \mathbf {p} \right)=\mathbf {L} \cdot \mathbf {L} =L^{2}}
所以,
A
r
cos
θ
=
L
2
−
m
k
r
{\displaystyle Ar\cos \theta =L^{2}-mkr}
編排成圓錐曲線 的方程式形式:
1
r
=
m
k
L
2
(
1
+
A
m
k
cos
θ
)
{\displaystyle {\frac {1}{r}}={\frac {mk}{L^{2}}}\left(1+{\frac {A}{mk}}\cos \theta \right)}
離心率
e
{\displaystyle e}
e
=
A
m
k
=
|
A
|
m
k
{\displaystyle e={\frac {A}{mk}}={\frac {\left|\mathbf {A} \right|}{mk}}}
克卜勒軌道與能量的關係可以由LRL向量推導出。
A
{\displaystyle \mathbf {A} }
A
⋅
A
=
(
p
×
L
−
m
k
r
^
)
⋅
(
p
×
L
−
m
k
r
^
)
=
p
2
L
2
+
m
2
k
2
−
2
m
k
r
^
⋅
(
p
×
L
)
=
(
2
m
E
+
2
m
k
r
)
L
2
+
m
2
k
2
−
2
m
k
r
L
2
{\displaystyle {\begin{aligned}\mathbf {A} \cdot \mathbf {A} &=(\mathbf {p} \times \mathbf {L} -mk\mathbf {\hat {r}} )\cdot (\mathbf {p} \times \mathbf {L} -mk\mathbf {\hat {r}} )\\&=p^{2}L^{2}+m^{2}k^{2}-2mk{\hat {\mathbf {r} }}\cdot (\mathbf {p} \times \mathbf {L} )\\&=\left(2mE+{\frac {2mk}{r}}\right)L^{2}+m^{2}k^{2}-{\frac {2mk}{r}}L^{2}\\\end{aligned}}}
。 所以,
A
2
=
m
2
k
2
+
2
m
E
L
2
{\displaystyle A^{2}=m^{2}k^{2}+2mEL^{2}}
稍微編排,離心率的平方
e
2
{\displaystyle e^{2}}
E
{\displaystyle E}
e
2
=
1
+
2
L
2
m
k
2
E
{\displaystyle e^{2}=1+{\frac {2L^{2}}{mk^{2}}}E}
假若能量
E
{\displaystyle E}
束縛軌道 ),則離心率小於1,這軌道是橢圓 形軌道。相反地,假若能量是正值的(非束縛軌道 ,又稱為散射軌道 )則離心率大於1,這軌道是雙曲線 軌道。最後,假若能量等於零,則離心率等於1,這軌道是拋物線 軌道。對於所有狀況,LRL向量與圓錐曲線的對稱軸平行,而且從力中心點 指向近拱點 。
圖3 :在平方反比連心力作用下,隨著粒子的軌道運動,使用速端曲線圖,固定動量向量
p
{\displaystyle \mathbf {p} }
y -軸,py -座標為
A
/
L
{\displaystyle A/L}
m
k
/
L
{\displaystyle mk/L}
假設一個粒子在做軌道運動。其速度向量的物理行為可以用速端曲線 顯示出來,而動量是速度乘以質量。所以,速端曲線也可以顯示出動量的物理行為。在平方反比連心力作用下,速端曲線(圖3)顯示出,粒子的動量向量的頭部呈圓形移動;這事實可以用LRL向量
A
{\displaystyle \mathbf {A} }
L
{\displaystyle \mathbf {L} }
[ 13] [ 6]
L
{\displaystyle \mathbf {L} }
A
{\displaystyle \mathbf {A} }
叉積 :
L
2
p
=
L
×
A
−
m
k
r
^
×
L
{\displaystyle L^{2}\mathbf {p} =\mathbf {L} \times \mathbf {A} -mk{\hat {\mathbf {r} }}\times \mathbf {L} }
設定xyz參考系的圓點在力中心點 ,
L
{\displaystyle \mathbf {L} }
半長軸 同軸。則
p
x
2
+
(
p
y
−
A
/
L
)
2
=
(
m
k
/
L
)
2
{\displaystyle p_{x}^{2}+\left(p_{y}-A/L\right)^{2}=\left(mk/L\right)^{2}}
換句話說,動量
p
{\displaystyle \mathbf {p} }
m
k
/
L
{\displaystyle mk/L}
(
0
,
A
/
L
)
{\displaystyle (0,\ A/L)}
克卜勒問題 的對稱性 。
夾角
η
{\displaystyle \eta }
y -軸。很顯然地,離心率等於
cos
η
{\displaystyle \cos \eta }
p
0
=
2
m
|
E
|
{\displaystyle p_{0}={\sqrt {2m\left|E\right|}}}
在克卜勒問題裏,兩個向量
A
{\displaystyle \mathbf {A} }
L
{\displaystyle \mathbf {L} }
E
{\displaystyle E}
A
⋅
L
=
0
{\displaystyle \mathbf {A} \cdot \mathbf {L} =0}
A
2
=
m
2
k
2
+
2
m
E
L
2
{\displaystyle A^{2}=m^{2}k^{2}+2mEL^{2}}
A
{\displaystyle \mathbf {A} }
L
{\displaystyle \mathbf {L} }
E
{\displaystyle E}
A
{\displaystyle \mathbf {A} }
L
{\displaystyle \mathbf {L} }
A
{\displaystyle \mathbf {A} }
由於有上述兩個關係公式,這物理系統一共有五個獨立的運動常數 。這結果與設定粒子軌道所需的六個初始條件(粒子的初始位置向量與初始速度向量,每一個向量有三個分量)相符合,原因是運動常數不涉及初始時間(視六個初始條件函數的參數為自變量 初始時間。用其中的一個初始條件函數除去這自變量;將此初始條件函數當作一個自變量,則剰餘五個初始條件函數,函數的參數為新自變量)。
因為運動方程式 是二階微分方程式,一個擁有
d
{\displaystyle d}
自由度 的物理系統,需要
2
d
{\displaystyle 2d}
初始條件 來設定解答。由於運動常數不涉及初始時間,這物理系統最多只能擁有
2
d
−
1
{\displaystyle 2d-1}
運動常數 。一個擁有超過
d
{\displaystyle d}
超級可積分系統 ;而一個擁有
2
d
−
1
{\displaystyle 2d-1}
最大超級可積分系統 [ 18] 哈密頓-亞可比方程式 的解答,採用任意一種坐標系統,最多只能求得
d
{\displaystyle d}
[ 19]
克卜勒問題擁有三個自由度(
d
=
3
{\displaystyle d=3}
球坐標 或拋物線坐標 ,哈密頓-亞可比方程式都是可積分的[ 20] 解釋 。最大超級可積分系統可以用對易關係 來量子化 ,這論據,稍後也會又更明瞭的說明 [ 21]
圖5:橢圓軌道的慢進動 ,離心率
e
=
0.667
{\displaystyle e=0.667}
進動 就會發生 只有在一個標準的平方反比 連心力 下,粒子的LRL向量
A
{\displaystyle \mathbf {A} }
平方反比定律 ,而可能會含有別種微擾 的連心力;稱其負值不定積分 為微擾勢 ,標記為
h
(
r
)
{\displaystyle h(r)}
慢進動 。假若微擾勢
h
(
r
)
{\displaystyle h(r)}
保守的 連心勢,也就是說,總能量
E
{\displaystyle E}
L
{\displaystyle \mathbf {L} }
L
{\displaystyle \mathbf {L} }
A
{\displaystyle A}
h
(
r
)
{\displaystyle h(r)}
h
(
r
)
=
−
h
r
n
{\displaystyle h(r)=-\ {\frac {h}{r^{n}}}}
其中,
h
{\displaystyle h}
n
≤
2
{\displaystyle n\leq 2}
用正則微擾理論 與作用量-角度座標 ,可以直接地推導出LRL向量的轉動率是[ 1]
Ω
¯
=
∂
∂
L
⟨
h
(
r
)
⟩
=
∂
∂
L
{
1
T
∫
0
T
h
(
r
)
d
t
}
=
∂
∂
L
{
m
T
L
∫
0
2
π
r
2
h
(
r
)
d
θ
}
{\displaystyle {\begin{aligned}{\bar {\Omega }}={\frac {\partial }{\partial L}}\langle h(r)\rangle &={\frac {\partial }{\partial L}}\left\{{\frac {1}{T}}\int _{0}^{T}h(r)\,\mathrm {d} t\right\}\\&={\frac {\partial }{\partial L}}\left\{{\frac {m}{TL}}\int _{0}^{2\pi }r^{2}h(r)\,\mathrm {d} \theta \right\}\\\end{aligned}}}
; 其中,
T
{\displaystyle T}
恆等式
L
d
t
=
m
r
2
d
θ
{\displaystyle Ldt=mr^{2}\mathrm {d} \theta }
⟨
h
(
r
)
⟩
{\displaystyle \langle h(r)\rangle }
這方法曾經被用來證實愛因斯坦 的廣義相對論 。廣義相對論在常見的牛頓萬有引力 項目外,又添加了一項小的反立方微擾[ 22]
h
(
r
)
=
k
L
2
m
2
c
2
(
1
r
3
)
{\displaystyle h(r)={\frac {kL^{2}}{m^{2}c^{2}}}\left({\frac {1}{r^{3}}}\right)}
將此函數代入積分。再代入
r
{\displaystyle r}
θ
{\displaystyle \theta }
1
r
=
m
k
L
2
(
1
+
A
m
k
cos
θ
)
{\displaystyle {\frac {1}{r}}={\frac {mk}{L^{2}}}\left(1+{\frac {A}{mk}}\cos \theta \right)}
就可以計算出這非牛頓微擾所產生的近拱點 進動率[ 22]
Ω
¯
=
6
π
k
2
T
L
2
c
2
{\displaystyle {\bar {\Omega }}={\frac {6\pi k^{2}}{TL^{2}c^{2}}}}
計算出的答案準確地符合實驗觀測到的水星 進動 數據[ 23] 雙重 脈衝星 數據[ 24] 廣義相對論 的強證[ 25] [ 26]
角動量
L
{\displaystyle \mathbf {L} }
L
i
{\displaystyle L_{i}}
帕松括號 是[ 1]
{
L
i
,
L
j
}
=
∑
s
=
1
3
ϵ
i
j
s
L
s
{\displaystyle \{L_{i},L_{j}\}=\sum _{s=1}^{3}\epsilon _{ijs}L_{s}}
其中,指標
i
,
j
=
1
,
2
,
3
{\displaystyle i,\ j=1,\ 2,\ 3}
直角座標系 的三個座標
(
x
,
y
,
z
)
{\displaystyle (x,\ y,\ z)}
ϵ
i
j
s
{\displaystyle \epsilon _{ijs}}
列維-奇維塔符號 ;在這裏,為了避免與力強度的標記
k
{\displaystyle k}
s
{\displaystyle s}
定義一個與LRL向量成比例的向量
D
{\displaystyle \mathbf {D} }
D
=
A
2
m
|
E
|
{\displaystyle \mathbf {D} ={\frac {\mathbf {A} }{\sqrt {2m\left|E\right|}}}}
向量
D
{\displaystyle \mathbf {D} }
L
{\displaystyle \mathbf {L} }
D
{\displaystyle \mathbf {D} }
L
{\displaystyle \mathbf {L} }
[ 27]
{
D
i
,
L
j
}
=
∑
s
=
1
3
ϵ
i
j
s
D
s
{\displaystyle \{D_{i},L_{j}\}=\sum _{s=1}^{3}\epsilon _{ijs}D_{s}}
向量
D
{\displaystyle \mathbf {D} }
E
{\displaystyle E}
E
{\displaystyle E}
雙曲線 軌道),或負值(在平方反比連心力作用下,產生閉合地橢圓 軌道)有關。假若總能量
E
{\displaystyle E}
{
D
i
,
D
j
}
=
−
∑
s
=
1
3
ϵ
i
j
s
L
s
{\displaystyle \{D_{i},D_{j}\}=-\sum _{s=1}^{3}\epsilon _{ijs}L_{s}}
反之,假若總能量
E
{\displaystyle E}
{
D
i
,
D
j
}
=
∑
s
=
1
3
ϵ
i
j
s
L
s
{\displaystyle \{D_{i},D_{j}\}=\sum _{s=1}^{3}\epsilon _{ijs}L_{s}}
由於以下這三個帕松括號方程式,
{
L
i
,
L
j
}
=
∑
s
=
1
3
ϵ
i
j
s
L
s
{\displaystyle \{L_{i},L_{j}\}=\sum _{s=1}^{3}\epsilon _{ijs}L_{s}}
{
D
i
,
L
j
}
=
∑
s
=
1
3
ϵ
i
j
s
D
s
{\displaystyle \{D_{i},L_{j}\}=\sum _{s=1}^{3}\epsilon _{ijs}D_{s}}
{
D
i
,
D
j
}
=
∑
s
=
1
3
ϵ
i
j
s
L
s
{\displaystyle \{D_{i},D_{j}\}=\sum _{s=1}^{3}\epsilon _{ijs}L_{s}}
如果總能量
E
{\displaystyle E}
旋轉群 SO(4)。
假若總能量
E
{\displaystyle E}
卡西米爾不變量
C
1
,
C
2
{\displaystyle C_{1},\ C_{2}}
C
1
=
D
⋅
D
+
L
⋅
L
=
m
k
2
2
|
E
|
{\displaystyle C_{1}=\mathbf {D} \cdot \mathbf {D} +\mathbf {L} \cdot \mathbf {L} ={\frac {mk^{2}}{2\left|E\right|}}}
C
2
=
D
⋅
L
=
0
{\displaystyle C_{2}=\mathbf {D} \cdot \mathbf {L} =0}
而且,卡西米爾不變量與
D
{\displaystyle \mathbf {D} }
{
C
1
,
D
i
}
=
{
C
2
,
D
i
}
=
0
{\displaystyle \{C_{1},D_{i}\}=\{C_{2},D_{i}\}=0}
還有,卡西米爾不變量與
L
{\displaystyle \mathbf {L} }
{
C
1
,
L
i
}
=
{
C
2
,
L
i
}
=
0
{\displaystyle \{C_{1},L_{i}\}=\{C_{2},L_{i}\}=0}
既然兩個向量
D
{\displaystyle \mathbf {D} }
L
{\displaystyle \mathbf {L} }
C
2
{\displaystyle C_{2}}
C
1
{\displaystyle C_{1}}
m
{\displaystyle m}
k
{\displaystyle k}
E
{\displaystyle E}
C
1
{\displaystyle C_{1}}
D
i
{\displaystyle D_{i}}
L
i
{\displaystyle L_{i}}
C
1
{\displaystyle C_{1}}
量子力學 的正則對易關係 ,就可以推導出類氫原子 的原子能級 ,而不必用到的薛丁格方程式 。
圖6:從LRL向量算符與角動量算符的對易關係,預測出來的氫原子的原子能級。各種實驗都準確地證實這些能級正確無誤。 帕松括號提供了一個簡易的方法來正則量子化 古典系統。兩個量子算符 的對易關係 等於
i
ℏ
{\displaystyle i\hbar }
[ 28] 卡西米爾算符
C
1
{\displaystyle C_{1}}
本徵值 ,沃爾夫岡·包利 成功地推導出類氫原子 的原子能級 (參閱圖6),以及其發射光譜 [ 3] 薛丁格方程式 成立之前[ 29]
LRL向量
A
{\displaystyle \mathbf {A} }
叉積 必須仔細地加以定義[ 27]
A
k
≡
−
m
e
α
r
^
k
+
1
2
∑
i
=
1
3
∑
j
=
1
3
ϵ
i
j
k
(
p
i
l
j
+
l
j
p
i
)
{\displaystyle A_{k}\equiv -m_{e}\alpha {\hat {r}}_{k}+{\frac {1}{2}}\sum _{i=1}^{3}\sum _{j=1}^{3}\epsilon _{ijk}\left(p_{i}l_{j}+l_{j}p_{i}\right)}
其中,
m
e
{\displaystyle m_{e}}
α
=
e
2
4
π
ϵ
0
{\displaystyle \alpha ={\frac {e^{2}}{4\pi \epsilon _{0}}}}
e
{\displaystyle e}
單位電荷量 ,
ϵ
0
{\displaystyle \epsilon _{0}}
真空電容率 。
這定義有一個特性:指標
i
,
j
{\displaystyle i,\ j}
i
,
j
{\displaystyle i,\ j}
A
k
{\displaystyle A_{k}}
A
=
−
m
e
α
r
^
+
1
2
(
p
×
L
−
L
×
p
)
{\displaystyle \mathbf {A} =-m_{e}\alpha {\hat {r}}+{\frac {1}{2}}(\mathbf {p} \times \mathbf {L} -\mathbf {L} \times \mathbf {p} )}
那麼,其對應的哈密頓算符 是
H
=
p
2
2
m
e
−
α
r
{\displaystyle H={\frac {\mathbf {p} ^{2}}{2m_{e}}}-{\frac {\alpha }{r}}}
與
A
{\displaystyle \mathbf {A} }
D
{\displaystyle \mathbf {D} }
D
=
A
−
2
m
e
H
{\displaystyle \mathbf {D} ={\frac {\mathbf {A} }{\sqrt {-2m_{e}H}}}}
請注意,由於哈密頓算符的本徵值是負值,所以公式內的平方根是個實數。
經過一番繁冗的運算,可以求得對易關係:
{
L
i
,
L
j
}
=
i
ℏ
ϵ
i
j
k
L
k
{\displaystyle \{L_{i},\,L_{j}\}=i\hbar \epsilon _{ijk}L_{k}}
{
L
i
,
D
j
}
=
i
ℏ
ϵ
i
j
k
D
k
{\displaystyle \{L_{i},\,D_{j}\}=i\hbar \epsilon _{ijk}D_{k}}
{
D
i
,
D
j
}
=
i
ℏ
ϵ
i
j
k
L
k
{\displaystyle \{D_{i},\,D_{j}\}=i\hbar \epsilon _{ijk}L_{k}}
{
H
,
D
i
}
=
0
{\displaystyle \{H,\,D_{i}\}=0}
定義第一階張量 算符 為
J
0
≡
D
3
{\displaystyle J_{0}\equiv D_{3}}
J
±
1
≡
∓
1
2
(
D
1
±
i
D
2
)
{\displaystyle J_{\pm 1}\equiv \mp {\frac {1}{\sqrt {2}}}\left(D_{1}\pm iD_{2}\right)}
一個歸一化 的第一卡西米爾算符可以同樣地定義為
C
1
≡
D
2
+
L
2
=
m
e
α
2
−
2
H
−
ℏ
2
{\displaystyle C_{1}\equiv \mathbf {D} ^{2}+\mathbf {L} ^{2}={\frac {m_{e}\alpha ^{2}}{-2H}}-\hbar ^{2}}
注意到
J
+
1
{\displaystyle J_{+1}}
J
−
1
{\displaystyle J_{-1}}
{
J
+
1
,
J
−
1
}
=
i
{
D
1
,
D
2
}
=
−
ℏ
L
3
{\displaystyle \{J_{+1},J_{-1}\}=i\{D_{1},\,D_{2}\}=-\hbar L_{3}}
應用維格納-埃卡特定理 (Wigner-Eckart theorem ),
J
0
|
l
,
m
⟩
=
i
l
2
−
m
2
C
l
|
l
−
1
,
m
⟩
−
i
(
l
+
1
)
2
−
m
2
C
l
+
1
|
l
+
1
,
m
⟩
{\displaystyle J_{0}|l,\,m\rangle =i{\sqrt {l^{2}-m^{2}}}\ {\mathfrak {C}}_{l}|l-1,\,m\rangle -i{\sqrt {(l+1)^{2}-m^{2}}}\ {\mathfrak {C}}_{l+1}|l+1,\,m\rangle }
J
+
1
|
l
,
m
⟩
=
−
i
(
l
−
m
)
(
l
−
m
−
1
)
/
2
C
l
|
l
−
1
,
m
+
1
⟩
−
i
(
l
+
m
+
1
)
(
l
+
m
+
2
)
/
2
C
l
+
1
|
l
+
1
,
m
+
1
⟩
{\displaystyle J_{+1}|l,\,m\rangle =-i{\sqrt {(l-m)(l-m-1)/2}}\ {\mathfrak {C}}_{l}|l-1,\,m+1\rangle -i{\sqrt {(l+m+1)(l+m+2)/2}}\ {\mathfrak {C}}_{l+1}|l+1,\,m+1\rangle }
J
−
1
|
l
,
m
⟩
=
−
i
(
l
+
m
)
(
l
+
m
−
1
)
/
2
C
l
|
l
−
1
,
m
−
1
⟩
−
i
(
l
−
m
+
1
)
(
l
−
m
+
2
)
/
2
C
l
+
1
|
l
+
1
,
m
−
1
⟩
{\displaystyle J_{-1}|l,\,m\rangle =-i{\sqrt {(l+m)(l+m-1)/2}}\ {\mathfrak {C}}_{l}|l-1,\,m-1\rangle -i{\sqrt {(l-m+1)(l-m+2)/2}}\ {\mathfrak {C}}_{l+1}|l+1,\,m-1\rangle }
其中,
|
l
,
m
⟩
{\displaystyle |l,\,m\rangle }
角量子數 為
l
{\displaystyle l}
磁量子數 為
l
{\displaystyle l}
本徵態 ,
C
l
{\displaystyle {\mathfrak {C}}_{l}}
經過一番運算,
J
+
1
{\displaystyle J_{+1}}
J
−
1
{\displaystyle J_{-1}}
|
l
,
m
⟩
{\displaystyle |l,\,m\rangle }
{
J
+
1
,
J
−
1
}
|
l
,
m
⟩
=
−
m
[
(
2
l
−
1
)
C
l
2
−
(
2
l
+
3
)
C
l
+
1
2
]
|
l
,
m
⟩
=
−
ℏ
L
3
|
l
,
m
⟩
=
−
m
ℏ
2
{\displaystyle {\begin{aligned}\{J_{+1},\,J_{-1}\}|l,\,m\rangle &=-m[(2l-1){\mathfrak {C}}_{l}^{2}-(2l+3){\mathfrak {C}}_{l+1}^{2}]|l,\,m\rangle \\&=-\hbar L_{3}|l,\,m\rangle =-m\hbar ^{2}\\\end{aligned}}}
。 所以,
C
l
{\displaystyle {\mathfrak {C}}_{l}}
遞迴關係 是
(
2
l
−
1
)
C
l
2
−
(
2
l
+
3
)
C
l
+
1
2
=
ℏ
2
{\displaystyle (2l-1){\mathfrak {C}}_{l}^{2}-(2l+3){\mathfrak {C}}_{l+1}^{2}=\hbar ^{2}}
假設
C
l
2
{\displaystyle {\mathfrak {C}}_{l}^{2}}
l
>
0
{\displaystyle l>0}
l
{\displaystyle l}
l
m
a
x
{\displaystyle l_{max}}
|
l
m
a
x
+
1
,
⟩
{\displaystyle |l_{max}+1,\,\ \rangle }
C
l
m
a
x
+
1
=
0
{\displaystyle {\mathfrak {C}}_{l_{max}+1}=0}
C
l
m
a
x
=
ℏ
2
2
l
m
a
x
−
1
{\displaystyle {\mathfrak {C}}_{l_{max}}={\frac {\hbar ^{2}}{2l_{max}-1}}}
n
=
l
m
a
x
−
1
{\displaystyle n=l_{max}-1}
C
l
{\displaystyle {\mathfrak {C}}_{l}}
C
l
=
n
2
−
l
2
4
l
2
−
1
ℏ
{\displaystyle {\mathfrak {C}}_{l}={\sqrt {\frac {n^{2}-l^{2}}{4l^{2}-1}}}\ \hbar }
這個
n
{\displaystyle n}
主量子數 。先計算
D
2
{\displaystyle D^{2}}
D
2
|
n
,
l
,
m
⟩
=
[
J
+
1
J
−
1
+
J
−
1
J
+
1
+
J
0
2
]
|
n
,
l
,
m
⟩
=
(
n
2
−
l
2
−
l
−
1
)
ℏ
2
|
n
,
l
,
m
⟩
{\displaystyle {\begin{aligned}D^{2}|n,\,l,\,m\rangle &=[J_{+1}J_{-1}+J_{-1}J_{+1}+J_{0}^{2}]|n,\,l,\,m\rangle \\&=(n^{2}-l^{2}-l-1)\hbar ^{2}|n,\,l,\,m\rangle \\\end{aligned}}}
。 所以,第一卡西米爾算符
C
1
{\displaystyle C_{1}}
|
n
,
l
,
m
⟩
{\displaystyle |n,\,l,\,m\rangle }
C
1
|
n
,
l
,
m
⟩
=
(
D
2
+
L
2
)
|
n
,
l
,
m
⟩
=
(
n
2
−
1
)
ℏ
2
|
n
,
l
,
m
⟩
{\displaystyle C_{1}|n,\,l,\,m\rangle =(D^{2}+L^{2})|n,\,l,\,m\rangle =(n^{2}-1)\hbar ^{2}|n,\,l,\,m\rangle }
第一卡西米爾算符
C
1
{\displaystyle C_{1}}
(
n
2
−
1
)
ℏ
2
{\displaystyle (n^{2}-1)\hbar ^{2}}
l
{\displaystyle l}
m
{\displaystyle m}
原子能階 的簡併 [ 27]
E
n
=
−
m
e
α
2
2
ℏ
2
n
2
=
−
m
e
e
4
2
n
2
(
4
π
ϵ
0
)
2
ℏ
2
{\displaystyle E_{n}=-{\frac {m_{e}\alpha ^{2}}{2\hbar ^{2}n^{2}}}=-{\frac {m_{e}e^{4}}{2n^{2}(4\pi \epsilon _{0})^{2}\hbar ^{2}}}}
這就是著名的氫原子 波耳公式 。
在克卜勒問題裏,LRL向量的保守性對應於系統的一種微妙的對稱性 。在古典力學 裏,對稱性可以由連續運算顯示出來;這連續運算可以將一個軌道映射 至另外一個軌道,而同時保持系統的能量不變。在量子力學 裏,連續運算將同能級 原子軌域 混合在一起,也就是說,(簡併原子能級 )。
通常,對於每一個對稱性都會存在有一個保守量[ 1] 連心力 系統必對稱於旋轉群 SO(3) ;因而指引出角動量
L
{\displaystyle \mathbf {L} }
古典力學 裏,整個系統的旋轉不會影響軌道的能量。在量子力學 裏,假若旋轉只混合角量子數 相同的球諧函數 ,則系統的能量不會改變。
圖7:同能量的動量的速端曲線家族。每一個圓圈都經過在px -軸上,同樣的兩點
±
p
0
=
±
2
m
|
E
|
{\displaystyle \pm p_{0}=\pm {\sqrt {2m\left|E\right|}}}
阿波羅尼奧斯圓 ,和雙極坐標 的
σ
{\displaystyle \sigma }
坐標曲面 。 平方反比連心力系統的對稱性是更高維與更微妙的。這奇特的對稱性是由角動量
L
{\displaystyle \mathbf {L} }
A
{\displaystyle \mathbf {A} }
氫原子 的能級跟角量子數
l
{\displaystyle l}
m
{\displaystyle m}
更高維空間 ,使得這對稱性更加的微妙;這類的對稱性常稱為隱祕對稱性 [ 30]
l
{\displaystyle l}
m
{\displaystyle m}
s
(
l
=
0
)
{\displaystyle s(l=0)}
p
(
l
=
1
)
{\displaystyle p(l=1)}
原子軌域 的混合。這種混合是不能用普通的三維平移運算或旋轉運算達成的。可是,這種混合等價於高維度空間的旋轉。
在一個束縛(bounded)系統裏,能量是負值的,這高維對稱群 是SO(4);特性是四維向量的長度保持不變:
|
e
|
2
=
e
1
2
+
e
2
2
+
e
3
2
+
e
4
2
{\displaystyle \left|\mathbf {e} \right|^{2}=e_{1}^{2}+e_{2}^{2}+e_{3}^{2}+e_{4}^{2}}
1935年,弗拉基米爾·福克 表明,在量子力學裏,束縛的克卜勒問題等價於一個粒子自由地移動於四維空間的三維單位球 [ 4] 薛丁格 波函數 是球諧函數 的球極平面投影 。圓球的旋轉與重複射影造成了橢圓軌域的連續映射 ,同時維持能量不變;這對應於主量子數
n
{\displaystyle n}
華倫泰·巴格曼 注意到,跟LRL向量成比例的向量
D
{\displaystyle \mathbf {D} }
L
{\displaystyle \mathbf {L} }
帕松括號 形成SO(4)的李代數 [ 5]
D
{\displaystyle \mathbf {D} }
L
{\displaystyle \mathbf {L} }
在一個非束縛(unbound),散射 系統裏,能量是正值的,對應的高維對稱群 是SO(3,1);其特性是保持四維向量 的閔考斯基長度 不變:
d
s
2
=
e
1
2
+
e
2
2
+
e
3
2
−
e
4
2
{\displaystyle ds^{2}=e_{1}^{2}+e_{2}^{2}+e_{3}^{2}-e_{4}^{2}}
連心力系統(包括克卜勒問題的那些系統)的軌道對於反射 也具有對稱性。所以,軌道的完全對稱群並不是前面所提的SO(3) 、SO(4)、SO(3,1)群;而分別是O(3) 、O(4) 、O(3,1)。然而,只需要連通 子群 SO(3)、SO(4)、SO(3,1)來展示出角動量與LRL向量的保守性;反射對稱性與保守性不相關。保守性可以由群的李代數 推導出來[ 31] [ 32]
圖8:圖7的動量的速端曲線對應於
η
{\displaystyle \eta }
三維單位球 的大圓線 的球極平面投影 。每一個大圓線都與
η
x
{\displaystyle \eta _{x}}
w
{\displaystyle w}
η
x
{\displaystyle \eta _{x}}
η
x
{\displaystyle \eta _{x}}
α
{\displaystyle \alpha }
離心率
e
=
s
i
n
α
{\displaystyle e=sin\ \alpha }
克卜勒問題 與四維旋轉對稱性SO(4)的關聯可以很容易地觀察出來[ 31] [ 33] [ 34] 直角座標 為
(
w
,
x
,
y
,
z
)
{\displaystyle (w,\ x,\ y,\ z)}
(
x
,
y
,
z
)
{\displaystyle (x,\ y,\ z)}
r
{\displaystyle \mathbf {r} }
p
{\displaystyle \mathbf {p} }
三維單位球 的四維向量
η
{\displaystyle {\boldsymbol {\eta }}}
η
=
p
2
−
p
0
2
p
2
+
p
0
2
w
^
+
2
p
0
p
2
+
p
0
2
p
{\displaystyle {\boldsymbol {\eta }}=\displaystyle {\frac {p^{2}-p_{0}^{2}}{p^{2}+p_{0}^{2}}}\mathbf {\hat {w}} +{\frac {2p_{0}}{p^{2}+p_{0}^{2}}}\mathbf {p} }
; 其中,
w
^
{\displaystyle \mathbf {\hat {w}} }
很簡單地,可以核對
η
{\displaystyle {\boldsymbol {\eta }}}
η
=
η
^
{\displaystyle {\boldsymbol {\eta }}={\hat {\boldsymbol {\eta }}}}
從
p
{\displaystyle \mathbf {p} }
η
^
{\displaystyle {\hat {\boldsymbol {\eta }}}}
映射 有一個獨特唯一的逆反;例如,動量
p
{\displaystyle \mathbf {p} }
p
x
=
p
0
η
x
1
−
η
w
{\displaystyle p_{x}=p_{0}{\frac {\eta _{x}}{1-\eta _{w}}}}
p
y
{\displaystyle p_{y}}
p
z
{\displaystyle p_{z}}
p
{\displaystyle \mathbf {p} }
η
^
{\displaystyle {\hat {\boldsymbol {\eta }}}}
球極平面投影 ,其比例因子為
p
0
{\displaystyle p_{0}}
選擇一個合適的直角座標,使z-軸與角動量
L
{\displaystyle \mathbf {L} }
取向 如同圖7,圓心包含於y-軸。這樣,不失廣義性,就可以觀察到這旋轉對稱性。由於粒子的運動包含於一個平面,
p
{\displaystyle \mathbf {p} }
L
{\displaystyle \mathbf {L} }
p
z
=
η
z
=
0
{\displaystyle p_{z}=\eta _{z}=0}
η
^
=
(
η
w
,
η
x
,
η
y
)
{\displaystyle {\hat {\boldsymbol {\eta }}}=(\eta _{w},\ \eta _{x},\ \eta _{y})}
阿波羅尼奧斯圓 家族對應於在三維單位球
η
{\displaystyle {\boldsymbol {\eta }}}
大圓線 家族。每一個大圓線與
η
x
{\displaystyle \eta _{x}}
η
x
=
±
1
{\displaystyle \eta _{x}=\pm 1}
p
x
=
±
p
0
{\displaystyle p_{x}=\pm p_{0}}
η
x
{\displaystyle \eta _{x}}
η
x
{\displaystyle \eta _{x}}
η
x
η
y
{\displaystyle \eta _{x}\eta _{y}}
α
{\displaystyle \alpha }
取任意一個大圓線
η
y
{\displaystyle \eta _{y}}
(
η
w
,
0
,
η
y
,
0
)
{\displaystyle (\eta _{w},\ 0,\ \eta _{y},\ 0)}
p
x
=
0
{\displaystyle p_{x}=0}
p
y
=
p
=
(
A
+
m
k
)
/
L
{\displaystyle p_{y}=p=(A+mk)/L}
η
y
=
cos
(
α
)
=
2
p
0
p
y
p
y
2
+
p
0
2
{\displaystyle \eta _{y}=\cos(\alpha )={\frac {2p_{0}p_{y}}{p_{y}^{2}+p_{0}^{2}}}}
經過一番運算,代入
p
0
{\displaystyle p_{0}}
sin
(
α
)
=
p
y
2
−
p
0
2
p
y
2
+
p
0
2
=
(
A
+
m
k
)
2
−
2
m
|
E
|
L
2
(
A
+
m
k
)
2
+
2
m
|
E
|
L
2
{\displaystyle {\begin{aligned}\sin(\alpha )&={\frac {p_{y}^{2}-p_{0}^{2}}{p_{y}^{2}+p_{0}^{2}}}\\&={\frac {(A+mk)^{2}-2m|E|L^{2}}{(A+mk)^{2}+2m|E|L^{2}}}\\\end{aligned}}}
。 給予一個束縛軌道,能量是負值的:
sin
(
α
)
=
(
A
+
m
k
)
2
+
2
m
E
L
2
(
A
+
m
k
)
2
−
2
m
E
L
2
=
A
m
k
=
e
{\displaystyle {\begin{aligned}\sin(\alpha )&={\frac {(A+mk)^{2}+2mEL^{2}}{(A+mk)^{2}-2mEL^{2}}}\\&={\frac {A}{mk}}=e\\\end{aligned}}}
。 所以,離心率
e
=
sin
(
α
)
{\displaystyle e=\sin(\alpha )}
α
{\displaystyle \alpha }
正弦函數 。
由於圖7的動量的速端曲線對應於
η
{\displaystyle \eta }
η
w
{\displaystyle \eta _{w}}
採用橢圓柱坐標
χ
,
ψ
,
ϕ
{\displaystyle \chi ,\ \psi ,\ \phi }
η
{\displaystyle {\boldsymbol {\eta }}}
作用量-角度座標 解答[ 35]
η
w
=
c
n
χ
c
n
ψ
{\displaystyle \eta _{w}=\mathrm {cn} \,\chi \ \mathrm {cn} \,\psi }
η
x
=
s
n
χ
d
n
ψ
cos
ϕ
{\displaystyle \eta _{x}=\mathrm {sn} \,\chi \ \mathrm {dn} \,\psi \ \cos \phi }
η
y
=
s
n
χ
d
n
ψ
sin
ϕ
{\displaystyle \eta _{y}=\mathrm {sn} \,\chi \ \mathrm {dn} \,\psi \ \sin \phi }
η
z
=
d
n
χ
s
n
ψ
{\displaystyle \eta _{z}=\mathrm {dn} \,\chi \ \mathrm {sn} \,\psi }
其中,
s
n
,
c
n
,
d
n
{\displaystyle \mathrm {sn} ,\,\mathrm {cn} ,\,\mathrm {dn} }
雅可比橢圓函數 。
以下幾種導引可以証明,在平方反比連心力下,LRL向量守恆。
假設,一個連心力
f
(
r
)
r
^
{\displaystyle f(\mathbf {r} ){\hat {\mathbf {r} }}}
牛頓第二定律 ,運動方程式為
d
p
d
t
=
f
(
r
)
r
^
{\displaystyle {\frac {\mathrm {d} \mathbf {p} }{\mathrm {d} t}}=f(\mathbf {r} ){\hat {\mathbf {r} }}}
其中,
f
(
r
)
{\displaystyle f(\mathbf {r} )}
r
{\displaystyle \mathbf {r} }
p
{\displaystyle \mathbf {p} }
t
{\displaystyle t}
由於在連心力下,角動量
L
=
r
×
p
{\displaystyle \mathbf {L} =\mathbf {r} \times \mathbf {p} }
d
d
t
L
=
0
{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} t}}\mathbf {L} =0}
所以,
d
d
t
(
p
×
L
)
=
d
p
d
t
×
L
=
f
(
r
)
r
^
×
(
r
×
m
d
r
d
t
)
=
f
(
r
)
m
r
[
r
(
r
⋅
d
r
d
t
)
−
r
2
d
r
d
t
]
{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} t}}\left(\mathbf {p} \times \mathbf {L} \right)={\frac {\mathrm {d} \mathbf {p} }{\mathrm {d} t}}\times \mathbf {L} =f(\mathbf {r} )\mathbf {\hat {r}} \times \left(\mathbf {r} \times m{\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}\right)=f(\mathbf {r} ){\frac {m}{r}}\left[\mathbf {r} \left(\mathbf {r} \cdot {\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}\right)-r^{2}{\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}\right]}
代入以下恆等式 :
r
⋅
d
r
d
t
=
1
2
d
d
t
(
r
⋅
r
)
=
1
2
d
d
t
(
r
2
)
=
r
d
r
d
t
{\displaystyle \mathbf {r} \cdot {\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}={\frac {1}{2}}{\frac {\mathrm {d} }{\mathrm {d} t}}\left(\mathbf {r} \cdot \mathbf {r} \right)={\frac {1}{2}}{\frac {\mathrm {d} }{\mathrm {d} t}}\left(r^{2}\right)=r{\frac {\mathrm {d} r}{\mathrm {d} t}}}
可以得到方程式,
d
d
t
(
p
×
L
)
=
−
m
f
(
r
)
r
2
[
1
r
d
r
d
t
−
r
r
2
d
r
d
t
]
=
−
m
f
(
r
)
r
2
d
d
t
(
r
r
)
{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} t}}\left(\mathbf {p} \times \mathbf {L} \right)=-mf(\mathbf {r} )r^{2}\left[{\frac {1}{r}}{\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}-{\frac {\mathbf {r} }{r^{2}}}{\frac {\mathrm {d} r}{\mathrm {d} t}}\right]=-mf(\mathbf {r} )r^{2}{\frac {\mathrm {d} }{\mathrm {d} t}}\left({\frac {\mathbf {r} }{r}}\right)}
代入平方反比連心力的方程式
f
(
r
)
=
−
k
r
2
{\displaystyle f(\mathbf {r} )={\frac {-k}{r^{2}}}}
d
d
t
(
p
×
L
)
=
m
k
d
d
t
(
r
r
)
=
d
d
t
(
m
k
r
^
)
{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} t}}\left(\mathbf {p} \times \mathbf {L} \right)=mk{\frac {\mathrm {d} }{\mathrm {d} t}}\left({\frac {\mathbf {r} }{r}}\right)={\frac {\mathrm {d} }{\mathrm {d} t}}\left(mk\mathbf {\hat {r}} \right)}
所以,在平方反比連心力下,
A
{\displaystyle \mathbf {A} }
d
d
t
A
=
d
d
t
(
p
×
L
)
−
d
d
t
(
m
k
r
^
)
=
0
{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} t}}\mathbf {A} ={\frac {\mathrm {d} }{\mathrm {d} t}}\left(\mathbf {p} \times \mathbf {L} \right)-{\frac {\mathrm {d} }{\mathrm {d} t}}\left(mk\mathbf {\hat {r}} \right)=0}
哈密頓-雅可比方程式 的可分性也可以用推導出LRL向量的恆定性[ 20] [ 36] 拋物線座標
(
ξ
,
η
)
{\displaystyle (\xi ,\ \eta )}
ξ
=
r
+
x
{\displaystyle \xi =r+x}
η
=
r
−
x
{\displaystyle \eta =r-x}
其中,
(
x
,
y
)
{\displaystyle (x,\ y)}
直角座標 ,
r
{\displaystyle r}
r
=
x
2
+
y
2
{\displaystyle r={\sqrt {x^{2}+y^{2}}}}
逆反過來,
x
=
1
2
(
ξ
−
η
)
{\displaystyle x={\frac {1}{2}}\left(\xi -\eta \right)}
y
=
ξ
η
{\displaystyle y={\sqrt {\xi \eta }}}
則克卜勒問題的哈密頓量 為
H
=
1
2
m
x
˙
2
+
1
2
m
y
˙
2
−
k
r
=
2
ξ
p
ξ
2
m
(
ξ
+
η
)
+
2
η
p
η
2
m
(
ξ
+
η
)
−
2
k
ξ
+
η
{\displaystyle {\begin{aligned}H&={\frac {1}{2}}m{\dot {x}}^{2}+{\frac {1}{2}}m{\dot {y}}^{2}-{\frac {k}{r}}\\&={\frac {2\xi p_{\xi }^{2}}{m(\xi +\eta )}}+{\frac {2\eta p_{\eta }^{2}}{m(\xi +\eta )}}-{\frac {2k}{\xi +\eta }}\\\end{aligned}}}
; 其中,
p
ξ
,
p
η
{\displaystyle p_{\xi },\ p_{\eta }}
廣義座標
ξ
,
η
{\displaystyle \xi ,\ \eta }
由於克卜勒問題的勢函數只跟廣義座標有關,哈密頓量是個能量運動常數,
H
=
E
{\displaystyle H=E}
2
ξ
p
ξ
2
−
m
k
−
m
E
ξ
=
−
2
η
p
η
2
+
m
k
+
m
E
η
{\displaystyle 2\xi p_{\xi }^{2}-mk-mE\xi =-2\eta p_{\eta }^{2}+mk+mE\eta }
這公式的左手邊與右手邊分別跟不同的廣義座標有關,所以,兩邊都相等於一個運動常數 ,標記為
Γ
{\displaystyle \Gamma }
2
ξ
p
ξ
2
−
m
k
−
m
E
ξ
=
−
Γ
{\displaystyle 2\xi p_{\xi }^{2}-mk-mE\xi =-\Gamma }
2
η
p
η
2
−
m
k
−
m
E
η
=
Γ
{\displaystyle 2\eta p_{\eta }^{2}-mk-mE\eta =\Gamma }
思考LRL向量的
x
{\displaystyle x}
A
x
=
p
y
(
x
p
y
−
y
p
x
)
−
m
k
x
r
=
x
p
y
2
−
y
p
x
p
y
−
m
k
+
m
η
k
r
{\displaystyle {\begin{aligned}A_{x}&=p_{y}(xp_{y}-yp_{x})-mk{\frac {x}{r}}\\&=xp_{y}^{2}-yp_{x}p_{y}-mk+m\eta {\frac {k}{r}}\\\end{aligned}}}
。 代入能量方程式
E
=
1
2
m
v
2
−
k
r
{\displaystyle E={\frac {1}{2}}mv^{2}-{\frac {k}{r}}}
A
x
=
x
p
y
2
−
y
p
x
p
y
+
1
2
m
2
v
2
η
−
m
k
−
m
E
η
{\displaystyle A_{x}=xp_{y}^{2}-yp_{x}p_{y}+{\frac {1}{2}}m^{2}v^{2}\eta -mk-mE\eta }
這公式右手邊,前三個項目,經過一番計算,可以得到
x
p
y
2
−
y
p
x
p
y
+
1
2
m
2
v
2
η
=
m
2
8
η
˙
2
(
η
+
ξ
)
2
η
=
2
η
p
η
2
{\displaystyle xp_{y}^{2}-yp_{x}p_{y}+{\frac {1}{2}}m^{2}v^{2}\eta ={\frac {m^{2}}{8}}{\dot {\eta }}^{2}{\frac {(\eta +\xi )^{2}}{\eta }}=2\eta p_{\eta }^{2}}
所以,
A
x
{\displaystyle A_{x}}
A
x
=
Γ
{\displaystyle A_{x}=\Gamma }
LRL向量的保守性與前面所提的旋轉對稱性,兩者之間的關係,可以用諾特定理 來做連結分析。諾特定理也可以用來辨明LRL向量是運動常數 。諾特定理表明[ 37] 廣義坐標
q
i
{\displaystyle q_{i}}
δ
q
i
=
ϵ
g
i
(
q
,
q
˙
,
t
)
{\displaystyle \delta q_{i}=\epsilon g_{i}(\mathbf {q} ,\ \mathbf {\dot {q}} ,\ t)}
ϵ
{\displaystyle \epsilon }
拉格朗日量
L
{\displaystyle {\mathcal {L}}}
變分
δ
L
{\displaystyle \delta {\mathcal {L}}}
δ
L
=
ϵ
d
d
t
G
(
q
,
t
)
{\displaystyle \delta {\mathcal {L}}=\epsilon {\frac {\mathrm {d} }{\mathrm {d} t}}G(\mathbf {q} ,\ t)}
則必存在保守量
Γ
{\displaystyle \Gamma }
Γ
=
−
G
+
∑
i
g
i
(
∂
L
∂
q
˙
i
)
{\displaystyle \Gamma =-G+\sum _{i}g_{i}\left({\frac {\partial {\mathcal {L}}}{\partial {\dot {q}}_{i}}}\right)}
其中,
g
i
(
q
,
q
˙
,
t
)
{\displaystyle g_{i}(\mathbf {q} ,\ \mathbf {\dot {q}} ,\ t)}
G
(
q
,
t
)
{\displaystyle G(\mathbf {q} ,\ t)}
更具體地,在一個克卜勒問題裏,試設定坐標
x
i
{\displaystyle x_{i}}
δ
x
i
=
ϵ
2
[
2
p
i
x
s
−
x
i
p
s
−
(
r
⋅
p
)
δ
i
s
]
{\displaystyle \delta x_{i}={\frac {\epsilon }{2}}\left[2p_{i}x_{s}-x_{i}p_{s}-(\mathbf {r} \cdot \mathbf {p} )\delta _{is}\right]}
其中,
i
=
1
,
2
,
3
{\displaystyle i=1,\ 2,\ 3}
x
i
{\displaystyle x_{i}}
p
i
{\displaystyle p_{i}}
r
{\displaystyle \mathbf {r} }
p
{\displaystyle \mathbf {p} }
i
{\displaystyle i}
δ
i
s
{\displaystyle \delta _{is}}
克羅內克爾δ ,
s
{\displaystyle s}
由於克卜勒問題的拉格朗日量是
L
=
∑
i
(
1
2
m
x
˙
i
x
˙
i
)
+
k
r
{\displaystyle {\mathcal {L}}=\sum _{i}\left({\frac {1}{2}}m{\dot {x}}_{i}{\dot {x}}_{i}\right)+{\frac {k}{r}}}
其運動方程式 為
m
x
¨
i
+
k
x
i
r
3
=
0
{\displaystyle m{\ddot {x}}_{i}+k{\frac {x_{i}}{r^{3}}}=0}
對應於坐標
x
i
{\displaystyle x_{i}}
x
˙
i
{\displaystyle {\dot {x}}_{i}}
δ
x
˙
i
=
ϵ
2
[
2
p
˙
i
x
s
−
x
i
p
˙
s
+
p
i
x
˙
s
−
p
2
m
δ
i
s
−
(
r
⋅
p
˙
)
δ
i
s
]
=
ϵ
2
[
−
k
r
3
x
i
x
s
+
p
i
x
˙
s
−
p
2
m
δ
i
s
+
k
r
δ
i
s
]
{\displaystyle {\begin{aligned}\delta {\dot {x}}_{i}&={\frac {\epsilon }{2}}\left[2{\dot {p}}_{i}x_{s}-x_{i}{\dot {p}}_{s}+p_{i}{\dot {x}}_{s}-{\frac {p^{2}}{m}}\delta _{is}-(\mathbf {r} \cdot {\dot {\mathbf {p} }})\delta _{is}\right]\\&={\frac {\epsilon }{2}}\left[-{\frac {k}{r^{3}}}x_{i}x_{s}+p_{i}{\dot {x}}_{s}-{\frac {p^{2}}{m}}\delta _{is}+{\frac {k}{r}}\delta _{is}\right]\\\end{aligned}}}
。 拉格朗日量取至一階的變分是
δ
L
=
∑
i
(
∂
L
∂
x
i
δ
x
i
+
∂
L
∂
x
˙
i
δ
x
˙
i
)
=
∑
i
(
−
k
x
i
r
3
δ
x
i
+
m
x
˙
i
δ
x
˙
i
)
{\displaystyle {\begin{aligned}\delta {\mathcal {L}}&=\sum _{i}\left({\frac {\partial {\mathcal {L}}}{\partial x_{i}}}\delta x_{i}+{\frac {\partial {\mathcal {L}}}{\partial {\dot {x}}_{i}}}\delta {\dot {x}}_{i}\right)\\&=\sum _{i}\left(-{\frac {kx_{i}}{r^{3}}}\delta x_{i}+m{\dot {x}}_{i}\delta {\dot {x}}_{i}\right)\\\end{aligned}}}
。 代入
δ
x
i
{\displaystyle \delta x_{i}}
δ
x
˙
i
{\displaystyle \delta {\dot {x}}_{i}}
δ
L
=
ϵ
m
k
d
d
t
(
x
s
r
)
{\displaystyle \delta {\mathcal {L}}=\epsilon mk{\frac {\mathrm {d} }{\mathrm {d} t}}\left({\frac {x_{s}}{r}}\right)}
再代入保守量
Γ
{\displaystyle \Gamma }
Γ
=
p
2
x
s
−
p
s
(
r
⋅
p
)
−
m
k
x
s
r
=
[
p
×
L
−
m
k
r
^
]
s
{\displaystyle \Gamma =p^{2}x_{s}-p_{s}\left(\mathbf {r} \cdot \mathbf {p} \right)-{\frac {mkx_{s}}{r}}=\left[\mathbf {p} \times \mathbf {L} -mk{\hat {\mathbf {r} }}\right]_{s}}
而這正是LRL向量的
s
{\displaystyle s}
A
s
{\displaystyle A_{s}}
圖9:推導出LRL向量保守性的李變換。當這比例參數
λ
{\displaystyle \lambda }
e
{\displaystyle e}
A
{\displaystyle \mathbf {A} }
諾特定理 精緻地推導出LRL向量的保守性。美中不足地,這導引有一個弱點:坐標變分
δ
x
i
{\displaystyle \delta x_{i}}
r
{\displaystyle \mathbf {r} }
p
{\displaystyle \mathbf {p} }
[ 38] 索菲斯·李 創建的方法來推導,可以除去這弱點[ 39] [ 40] 李變換 [ 30]
r
{\displaystyle \mathbf {r} }
t
{\displaystyle t}
λ
{\displaystyle \lambda }
t
→
λ
3
t
,
r
→
λ
2
r
,
p
→
1
λ
p
{\displaystyle t\rightarrow \lambda ^{3}t,\ \mathbf {r} \rightarrow \lambda ^{2}\mathbf {r} ,\ \mathbf {p} \rightarrow {\frac {1}{\lambda }}\mathbf {p} }
這變換改變了角動量
L
{\displaystyle L}
E
{\displaystyle E}
L
→
λ
L
,
E
→
1
λ
2
E
{\displaystyle L\rightarrow \lambda L,\ E\rightarrow {\frac {1}{\lambda ^{2}}}E}
可是,仍舊保持乘積
E
L
2
{\displaystyle EL^{2}}
e
{\displaystyle e}
A
{\displaystyle \mathbf {A} }
A
2
{\displaystyle A^{2}}
A
2
=
m
2
k
2
e
2
=
m
2
k
2
+
2
m
E
L
2
{\displaystyle A^{2}=m^{2}k^{2}e^{2}=m^{2}k^{2}+2mEL^{2}}
由於半短軸 與半長軸 的取向不因整體的比例變換而改變,LRL向量
A
{\displaystyle \mathbf {A} }
克卜勒第三定律 也仍舊成立:半長軸
a
{\displaystyle a}
T
{\displaystyle T}
T
2
/
a
3
{\displaystyle {T^{2}}/{a^{3}}}
LRL向量可以推廣至其他狀況;可以用來辨認在其他狀況下的保守值。
假設,一個物理系統裏,存在著電場
E
{\displaystyle \mathbf {E} }
A
{\displaystyle {\mathcal {A}}}
[ 20] [ 41]
A
=
A
+
m
q
2
[
(
r
×
E
)
×
r
]
{\displaystyle {\mathcal {A}}=\mathbf {A} +{\frac {mq}{2}}\left[\left(\mathbf {r} \times \mathbf {E} \right)\times \mathbf {r} \right]}
其中,
q
{\displaystyle q}
電荷量 。
最廣義的LRL向量的形式可以表達為[ 8]
A
=
(
∂
ξ
∂
u
)
(
p
×
L
)
+
[
ξ
−
u
(
∂
ξ
∂
u
)
]
L
2
r
^
{\displaystyle {\mathcal {A}}=\left({\frac {\partial \xi }{\partial u}}\right)\left(\mathbf {p} \times \mathbf {L} \right)+\left[\xi -u\left({\frac {\partial \xi }{\partial u}}\right)\right]L^{2}\mathbf {\hat {r}} }
其中,
u
=
1
r
{\displaystyle u={\frac {1}{r}}}
伯特蘭定理 ),
ξ
=
cos
θ
{\displaystyle \xi =\cos \theta }
θ
{\displaystyle \theta }
θ
=
L
∫
u
d
u
m
2
c
2
(
γ
2
−
1
)
−
L
2
u
2
{\displaystyle \theta =L\int ^{u}{\frac {du}{\sqrt {m^{2}c^{2}\left(\gamma ^{2}-1\right)-L^{2}u^{2}}}}}
其中,
γ
{\displaystyle \gamma }
勞侖茲因子 。
如同前面所提,計算
L
{\displaystyle \mathbf {L} }
A
{\displaystyle {\mathcal {A}}}
副法線向量
B
{\displaystyle {\mathcal {B}}}
B
=
L
×
A
{\displaystyle {\mathcal {B}}=\mathbf {L} \times {\mathcal {A}}}
綜和兩個向量成為一個保守的並矢張量
W
{\displaystyle {\mathcal {W}}}
W
=
α
A
⊗
A
+
β
B
⊗
B
{\displaystyle {\mathcal {W}}=\alpha {\mathcal {A}}\otimes {\mathcal {A}}+\beta \,{\mathcal {B}}\otimes {\mathcal {B}}}
舉例說明,計算一個非相對論性,均向性諧振子 的LRL向量。由於作用力是連心力 ,
F
(
r
)
=
−
k
r
{\displaystyle \mathbf {F} (r)=-k\mathbf {r} }
P
{\displaystyle \mathbf {P} }
L
{\displaystyle \mathbf {L} }
並矢張量 可以表達為一個簡單的形式:
W
=
1
2
m
p
⊗
p
+
k
2
r
⊗
r
{\displaystyle {\mathcal {W}}={\frac {1}{2m}}\mathbf {p} \otimes \mathbf {p} +{\frac {k}{2}}\,\mathbf {r} \otimes \mathbf {r} }
其相應的LRL向量必較複雜
A
=
1
m
r
2
ω
0
A
−
m
r
2
E
+
L
2
{
(
p
×
L
)
+
(
m
r
ω
0
A
−
m
r
E
)
r
^
}
{\displaystyle {\mathcal {A}}={\frac {1}{\sqrt {mr^{2}\omega _{0}A-mr^{2}E+L^{2}}}}\left\{\left(\mathbf {p} \times \mathbf {L} \right)+\left(mr\omega _{0}A-mrE\right)\mathbf {\hat {r}} \right\}}
其中,
ω
0
=
k
m
{\displaystyle \omega _{0}={\sqrt {\frac {k}{m}}}}
不同於動量與角動量,並沒有學術界一致認同的LRL向量定義;在科學文獻裏,存在有幾種不同的比例因子與符號。前面所述的定義是最普遍的定義。另外一種常見的定義,將
A
{\displaystyle \mathbf {A} }
m
k
{\displaystyle mk}
離心率向量
e
{\displaystyle \mathbf {e} }
e
=
1
m
k
(
p
×
L
)
−
r
^
=
m
k
(
v
×
L
)
−
r
^
{\displaystyle \mathbf {e} ={\frac {1}{mk}}\left(\mathbf {p} \times \mathbf {L} \right)-\mathbf {\hat {r}} ={\frac {m}{k}}\left(\mathbf {v} \times \mathbf {L} \right)-\mathbf {\hat {r}} }
其中,
v
{\displaystyle \mathbf {v} }
離心率向量
e
{\displaystyle \mathbf {e} }
A
{\displaystyle \mathbf {A} }
離心率 。
別種比例的版本也可能會用到。例如,將
A
{\displaystyle \mathbf {A} }
m
{\displaystyle m}
M
=
v
×
L
−
k
r
^
{\displaystyle \mathbf {M} =\mathbf {v} \times \mathbf {L} -k\mathbf {\hat {r}} }
或者,將
A
{\displaystyle \mathbf {A} }
P
0
{\displaystyle P_{0}}
D
=
A
P
0
=
1
2
m
|
E
|
{
p
×
L
−
m
k
r
^
}
{\displaystyle \mathbf {D} ={\frac {\mathbf {A} }{P_{0}}}={\frac {1}{\sqrt {2m\left|E\right|}}}\left\{\mathbf {p} \times \mathbf {L} -mk\mathbf {\hat {r}} \right\}}
D
{\displaystyle \mathbf {D} }
L
{\displaystyle \mathbf {L} }
運動常數 的事實。
圖4:角動量
L
{\displaystyle \mathbf {L} }
A
{\displaystyle \mathbf {A} }
B
{\displaystyle \mathbf {B} }
A
{\displaystyle \mathbf {A} }
B
{\displaystyle \mathbf {B} }
另外一個保守的向量是副法線向量
B
{\displaystyle \mathbf {B} }
威廉·哈密頓 曾經研究過這向量[ 7]
B
=
p
−
(
m
k
L
2
r
)
(
L
×
r
)
{\displaystyle \mathbf {B} =\mathbf {p} -\left({\frac {mk}{L^{2}r}}\right)\ \left(\mathbf {L} \times \mathbf {r} \right)}
這保守的向量與橢圓的半短軸同直線。
A
{\displaystyle \mathbf {A} }
B
{\displaystyle \mathbf {B} }
叉積
L
{\displaystyle \mathbf {L} }
A
{\displaystyle \mathbf {A} }
B
{\displaystyle \mathbf {B} }
並矢張量
W
{\displaystyle {\mathcal {W}}}
[ 8]
W
=
α
A
⊗
A
+
β
B
⊗
B
{\displaystyle {\mathcal {W}}=\alpha \mathbf {A} \otimes \mathbf {A} +\beta \,\mathbf {B} \otimes \mathbf {B} }
其中,
α
{\displaystyle \alpha }
β
{\displaystyle \beta }
⊗
{\displaystyle \otimes }
張量積 。展開這公式為
W
i
j
=
α
A
i
A
j
+
β
B
i
B
j
{\displaystyle {\mathcal {W}}_{ij}=\alpha A_{i}A_{j}+\beta B_{i}B_{j}}
由於兩個向量互相垂直,
A
{\displaystyle \mathbf {A} }
B
{\displaystyle \mathbf {B} }
W
{\displaystyle {\mathcal {W}}}
主軸 ,也就是說,按比例的特徵向量 。由於
A
{\displaystyle \mathbf {A} }
B
{\displaystyle \mathbf {B} }
L
{\displaystyle \mathbf {L} }
W
{\displaystyle {\mathcal {W}}}
L
{\displaystyle \mathbf {L} }
L
⋅
W
=
α
(
L
⋅
A
)
A
+
β
(
L
⋅
B
)
B
=
0
{\displaystyle \mathbf {L} \cdot {\mathcal {W}}=\alpha \left(\mathbf {L} \cdot \mathbf {A} \right)\mathbf {A} +\beta \left(\mathbf {L} \cdot \mathbf {B} \right)\mathbf {B} =0}
^ 1.0 1.1 1.2 1.3 1.4 1.5 1.6 Goldstein, H. Classical Mechanics 2nd edition. Addison Wesley. 1980: 102 –105,410–422,536–538. ^ 阿諾爾德, 弗拉基米爾 . Mathematical Methods of Classical Mechanics, 2nd ed.. New York: Springer-Verlag. 1989: 38. ISBN 0-387-96890-3 . ^ 3.0 3.1 3.2 包立, 沃爾夫岡 , Über das Wasserstoffspektrum vom Standpunkt der neuen Quantenmechanik, Zeitschrift für Physik, 1926, 36 : 336–363 ^ 4.0 4.1 Fock, V. , Zur Theorie des Wasserstoffatoms, Zeitschrift für Physik, 1935, 98 : 145–154 ^ 5.0 5.1 巴格曼, 華倫泰 , Zur Theorie des Wasserstoffatoms: Bemerkungen zur gleichnamigen Arbeit von V. Fock, Zeitschrift für Physik, 1936, 99 : 576–582 ^ 6.0 6.1 6.2 Goldstein, H., Prehistory of the Runge–Lenz vector, American Journal of Physics, 1975, 43 : 735–738 Goldstein, H., More on the prehistory of the Runge–Lenz vector, American Journal of Physics, 1976, 44 : 1123–1124 ^ 7.0 7.1 7.2 7.3 哈密頓, 威廉 , Applications of Quaternions to Some Dynamical Questions, Proceedings of the Royal Irish Academy, 1847, 3 : Appendix III ^ 8.0 8.1 8.2 Fradkin, D. M., Existence of the Dynamic Symmetries O4 and SU3 for All Classical Central Potential Problems, Progress of Theoretical Physics, 1967, 37 : 798–812 ^ Yoshida, T, Two methods of generalisation of the Laplace–Runge–Lenz vector, European Journal of Physics, 1987, 8 : 258–259 ^ 赫爾曼, 雅各布 , Unknown title, Giornale de Letterati D'Italia, 1710, 2 : 447–467 赫爾曼, 雅各布 , Extrait d'une lettre de M. Herman à M. Bernoulli datée de Padoüe le 12. Juillet 1710, Histoire de l'academie royale des sciences (Paris), 1710, 1732 : 519–521 ^ 白努利, 約翰 , Extrait de la Réponse de M. Bernoulli à M. Herman datée de Basle le 7. Octobre 1710, Histoire de l'academie royale des sciences (Paris), 1710, 1732 : 521–544 ^ 拉普拉斯, 皮埃爾-西蒙 . Traité de mécanique celeste. 1799: Tome I, Premiere Partie, Livre II, pp.165ff. ^ 13.0 13.1 哈密頓, 威廉 , The hodograph or a new method of expressing in symbolic language the Newtonian law of attraction, Proceedings of the Royal Irish Academy, 1847, 3 : 344–353 ^ 吉布斯, 約西亞 ; Wilson E. B. Vector Analysis. New York: Scribners. 1901: p. 135. ^ 龍格, 卡爾 . Vektoranalysis. Leipzig: Hirzel. 1919: Volume I. ^ 冷次, 威爾漢 , Über den Bewegungsverlauf und Quantenzustände der gestörten Keplerbewegung, Zeitschrift für Physik, 1924, 24 : 197–207 ^ Taff, L. G. Celestial Mechanics: A Computational Guide for the Practitioner. New York: John Wiley and Sons. 1985: 42–43. ^ Evans, N. W., Superintegrability in classical mechanics, Physical Review A, 1990, 41 : 5666–5676 ^ 索末菲, 阿諾 . Atomic Structure and Spectral Lines. London: Methuen. 1923: 118. ^ 20.0 20.1 20.2 朗道, 列夫 ; Lifshitz E. M. Mechanics 3rd edition. Pergamon Press. 1976: p. 154. ISBN 0-08-021022-8 (hardcover) and ISBN 0-08-029141-4 (softcover). ^ Evans, N. W., Group theory of the Smorodinsky–Winternitz system, Journal of Mathematical Physics, 1991, 32 : 3369–3375 ^ 22.0 22.1 愛因斯坦, 阿爾伯特 , Erklärung der Perihelbewegung des Merkur aus der allgemeinen Relativitätstheorie, Sitzungsberichte der Preussischen Akademie der Wissenschaften, 1915, 1915 : 831–839 ^ Le Verrier , Lettre de M. Le Verrier à M. Faye sur la Théorie de Mercure et sur le Mouvement du Périhélie de cette Planète, Comptes Rendus de l'Academie de Sciences (Paris), 1859, 49 : 379–383 ^ Will, C. M. General Relativity, an Einstein Century Survey SW Hawking and W Israel, eds. Cambridge: Cambridge University Press. 1979: Chapter 2. ^ 派斯, 亞伯拉罕 . Subtle is the Lord: The Science and the Life of Albert Einstein. Oxford University Press. 1982. ^ Roseveare, N. T. Mercury's Perihelion from Le Verrier to Einstein. Oxford University Press. 1982. ^ 27.0 27.1 27.2 Bohm, A. Quantum Mechanics: Foundations and Applications 2nd edition. Springer Verlag. 1986: 208 –222. ^ 狄拉克, 保羅 . Principles of Quantum Mechanics, 4th revised edition. Oxford University Press. 1958. ^ 薛丁格, 埃爾文 , Quantisierung als Eigenwertproblem, Annalen der Physik, 1926, 384 : 361–376 ^ 30.0 30.1 Prince, GE; Eliezer CJ, On the Lie symmetries of the classical Kepler problem, Journal of Physics A: Mathematical and General, 1981, 14 : 587–596 ^ 31.0 31.1 Bander, M; Itzykson C, Group Theory and the Hydrogen Atom (I), Reviews of Modern Physics, 1966, 38 : 330–345 ^ Bander, M; Itzykson C, Group Theory and the Hydrogen Atom (II), Reviews of Modern Physics, 1966, 38 : 346–358 ^ Rogers, HH, Symmetry transformations of the classical Kepler problem, Journal of Mathematical Physics, 1973, 14 : 1125–1129 ^ Guillemin, V; Sternberg S. Variations on a Theme by Kepler . American Mathematical Society Colloquium Publications, volume 42. 1990. ISBN 0-8218-1042-1 . ^ Lakshmanan, M; Hasegawa H, On the canonical equivalence of the Kepler problem in coordinate and momentum spaces, Journal of Physics A: L889–L893 ^ Dulock, VA; McIntosh HV, On the Degeneracy of the Kepler Problem, Pacific Journal of Mathematics, 1966, 19 : 39–55 ^ Lévy-Leblond, JM, Conservation Laws for Gauge-Invariant Lagrangians in Classical Mechanics, American Journal of Physics, 1971, 39 : 502–506 ^ Gonzalez-Gascon, F, Notes on the symmetries of systems of differential equations, Journal of Mathematical Physics, 1977, 18 : 1763–1767 ^ 李, 索菲斯 . Vorlesungen über Differentialgleichungen. Leipzig: Teubner. 1891. ^ Ince, EL. Ordinary Differential Equations. New York: Dover (1956 reprint). 1926: 93–113. ^ Redmond, P. J., Generalization of the Runge–Lenz Vector in the Presence of an Electric Field, Physical Review, 1964, 133 : B1352–B1353
Leach, P.G.L.; G.P. Flessas, Generalisations of the Laplace–Runge–Lenz vector, J. Nonlinear Math. Phys., 2003, 10 : 340–423,

















































































































![{\displaystyle {\begin{aligned}\{J_{+1},\,J_{-1}\}|l,\,m\rangle &=-m[(2l-1){\mathfrak {C}}_{l}^{2}-(2l+3){\mathfrak {C}}_{l+1}^{2}]|l,\,m\rangle \\&=-\hbar L_{3}|l,\,m\rangle =-m\hbar ^{2}\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/844f6c9eb531cf97779cc113427ad90f87abc09b)











![{\displaystyle {\begin{aligned}D^{2}|n,\,l,\,m\rangle &=[J_{+1}J_{-1}+J_{-1}J_{+1}+J_{0}^{2}]|n,\,l,\,m\rangle \\&=(n^{2}-l^{2}-l-1)\hbar ^{2}|n,\,l,\,m\rangle \\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6b11aedb31d3da0ceaa1e7beb862334c35c60338)

















































![{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} t}}\left(\mathbf {p} \times \mathbf {L} \right)={\frac {\mathrm {d} \mathbf {p} }{\mathrm {d} t}}\times \mathbf {L} =f(\mathbf {r} )\mathbf {\hat {r}} \times \left(\mathbf {r} \times m{\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}\right)=f(\mathbf {r} ){\frac {m}{r}}\left[\mathbf {r} \left(\mathbf {r} \cdot {\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}\right)-r^{2}{\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/936371c73c7d3c5aa64ce9cce60acfa9ad3e0c18)

![{\displaystyle {\frac {\mathrm {d} }{\mathrm {d} t}}\left(\mathbf {p} \times \mathbf {L} \right)=-mf(\mathbf {r} )r^{2}\left[{\frac {1}{r}}{\frac {\mathrm {d} \mathbf {r} }{\mathrm {d} t}}-{\frac {\mathbf {r} }{r^{2}}}{\frac {\mathrm {d} r}{\mathrm {d} t}}\right]=-mf(\mathbf {r} )r^{2}{\frac {\mathrm {d} }{\mathrm {d} t}}\left({\frac {\mathbf {r} }{r}}\right)}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4414eb629de2e805e7a71bc5e445f5764d7f3361)



































![{\displaystyle \delta x_{i}={\frac {\epsilon }{2}}\left[2p_{i}x_{s}-x_{i}p_{s}-(\mathbf {r} \cdot \mathbf {p} )\delta _{is}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6d576618d8d95e8b107f68fad05752a6f43b469a)







![{\displaystyle {\begin{aligned}\delta {\dot {x}}_{i}&={\frac {\epsilon }{2}}\left[2{\dot {p}}_{i}x_{s}-x_{i}{\dot {p}}_{s}+p_{i}{\dot {x}}_{s}-{\frac {p^{2}}{m}}\delta _{is}-(\mathbf {r} \cdot {\dot {\mathbf {p} }})\delta _{is}\right]\\&={\frac {\epsilon }{2}}\left[-{\frac {k}{r^{3}}}x_{i}x_{s}+p_{i}{\dot {x}}_{s}-{\frac {p^{2}}{m}}\delta _{is}+{\frac {k}{r}}\delta _{is}\right]\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/981d787295666d54338a8c532c0ae083c05a11da)




![{\displaystyle \Gamma =p^{2}x_{s}-p_{s}\left(\mathbf {r} \cdot \mathbf {p} \right)-{\frac {mkx_{s}}{r}}=\left[\mathbf {p} \times \mathbf {L} -mk{\hat {\mathbf {r} }}\right]_{s}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f6ef928dbc05f2d2e0f957970239c5825369a218)












![{\displaystyle {\mathcal {A}}=\mathbf {A} +{\frac {mq}{2}}\left[\left(\mathbf {r} \times \mathbf {E} \right)\times \mathbf {r} \right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7580676c243ced8662d593f4ed5501154e3e120c)

![{\displaystyle {\mathcal {A}}=\left({\frac {\partial \xi }{\partial u}}\right)\left(\mathbf {p} \times \mathbf {L} \right)+\left[\xi -u\left({\frac {\partial \xi }{\partial u}}\right)\right]L^{2}\mathbf {\hat {r}} }](https://wikimedia.org/api/rest_v1/media/math/render/svg/bb741e61862a023b299aa91f564340970424eb29)
























