卡马萨-霍尔姆方程

卡马萨-霍尔姆方程(Camassa Holm equation)是流体力学中的一个非线性偏微分方程

$u_{t}+2\kappa u_{x}-u_{xxt}+3uu_{x}=2u_{x}u_{xx}+uu_{xxx}.\,$

1993年卡马萨和霍尔姆以此偏微分方程模拟浅水波，

其中κ是大于0的参数。

行波解

卡马萨-霍尔姆方程3D动画

卡马萨-霍尔姆方程有行波解：

$u2:=(3/2)*{\frac {c*(c-2*\kappa )}{(cosh((1/2)*{\sqrt {((c-2*\kappa )/c)}}*(-x-x0+c*t))^{2}*(\kappa +c))}}$

参数：c = 1, x0 = 1, kappa = .3 代人得：

$u(x,t)={\frac {.463}{cosh(-.316*x-.316+.316*t)^{2}}}$

Maple TWSolution

Maple软件包TWSolution可提供多种行波解。

sech 展开

$g[2]:={u(x,t)=-(1/2)*kappa+_{C}5*sech(_{C}1+(1/2)*sqrt(3)*x-(1/4)*kappa*sqrt(3)*t)^{2}}$ $g[3]:={u(x,t)=-(1/2)*kappa+_{C}5*sech(_{C}1-(1/2)*sqrt(3)*x+(1/4)*kappa*sqrt(3)*t)^{2}}$ $g[4]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sech(_{C}1+(1/2*I)*sqrt(3)*x-(1/4*I)*kappa*sqrt(3)*t)^{2}}$ $g[5]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sech(_{C}1-(1/2*I)*sqrt(3)*x+(1/4*I)*kappa*sqrt(3)*t)^{2}}$ $g[6]:={u(x,t)=-(_{C}3-4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(3+4*_{C}2^{2}))+24*_{C}2*(2*_{C}3+kappa*_{C}2)*sech(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}$

• 
  Camassa Holm equation traveling wave sech plot5
• 
  Camassa Holm equation traveling wave sech plot4
• 
  Camassa Holm equation traveling wave sech plot6

exp 展开

$g[2]:={u(x,t)=-(1/9)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}5*exp(_{C}1-sqrt(3)*x+_{C}3*t)}$ $g[3]:={u(x,t)=(1/9)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}5*exp(_{C}1+sqrt(3)*x+_{C}3*t)}$ $g[5]:={u(x,t)=-(1/3)*sqrt(3)*_{C}3-(1/3)*kappa+_{C}7*(exp(_{C}1-(1/3)*sqrt(3)*x+_{C}3*t))^{3}}$

• 
  Camassa Holm equation traveling wave exp plot2
• 
  Camassa Holm equation traveling wave exp plot3
• 
  Camassa Holm equation traveling wave exp plot5

csch 展开

$g[2]:={u(x,t)=-(1/2)*kappa+_{C}5*csch(_{C}1+(1/2)*sqrt(3)*x-(1/4)*kappa*sqrt(3)*t)^{2}}$ $g[4]:={u(x,t)=_{C}4+((3/2)*_{C}4+(3/4)*kappa)*csch(_{C}1+(1/2*I)*sqrt(3)*x-(1/4*I)*kappa*sqrt(3)*t)^{2}}$ $g[6]:={u(x,t)=-(_{C}3-4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(4*_{C}2^{2}+3))-24*_{C}2*(2*_{C}3+kappa*_{C}2)*csch(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}$

• 
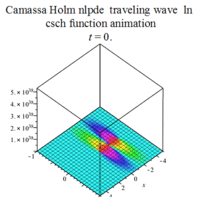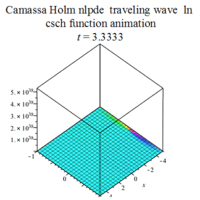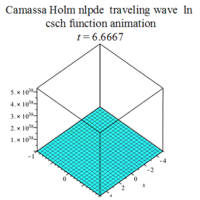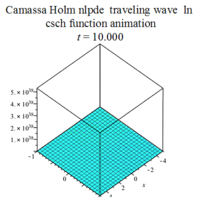
  Camassa Holm equation traveling wave csch plot2
• 
  Camassa Holm equation traveling wave csch plot4
• 
  Camassa Holm equation traveling wave csch plot6

sec 展开

$g[3]:={u(x,t)=-(1/2)*kappa+_{C}5*sec(_{C}1-(1/2*I)*sqrt(3)*x+(1/4*I)*kappa*sqrt(3)*t)^{2}}$ $g[5]:={u(x,t)=_{C}4+(-(3/2)*_{C}4-(3/4)*kappa)*sec(_{C}1-(1/2)*sqrt(3)*x+(1/4)*kappa*sqrt(3)*t)^{2}}$

$g[6]:={u(x,t)=(_{C}3+4*_{C}3*_{C}2^{2}+2*kappa*_{C}2)/(_{C}2*(4*_{C}2^{2}-3))-24*_{C}2*(2*_{C}3+kappa*_{C}2)*sec(_{C}1+_{C}2*x+_{C}3*t)^{2}/(16*_{C}2^{4}-9)}$

• 
  Camassa Holm equation traveling wave sec plot3
• 
  Camassa Holm equation traveling wave sec plot5

JacobiSN 展开

$g[3]:={u(x,t)=(1/9*I)*sqrt(3)*_{C}4-(1/3)*kappa+_{C}6*sin(_{C}2-I*sqrt(3)*x+_{C}4*t)}$ $g[4]:={u(x,t)=-(2/9*I)*sqrt(3)*_{C}4-(1/2)*_{C}7-(1/3)*kappa+_{C}7*sin(_{C}2+(1/2*I)*sqrt(3)*x+_{C}4*t)^{2}}$

Camassa Holm equation traveling wave Jacobish plot4

${\displaystyle }$ ${\displaystyle }$ ${\displaystyle }$ ${\displaystyle }$ ${\displaystyle }$

参考文献

• Beals, Richard; Sattinger, David H.; Szmigielski, Jacek, Multi-peakons and a theorem of Stieltjes, Inverse Problems 15 (1), 1999, 15 (1): L1–L4, Bibcode:1999InvPr..15L...1B, arXiv:solv-int/9903011 , doi:10.1088/0266-5611/15/1/001 
• Boldea, Costin-Radu, A generalization for peakon's solitary wave and Camassa–Holm equation, General Mathematics 5 (1–4), 1995, 5 (1–4): 33–42 [2013-12-30], （原始内容存档于2020-07-06） 
• Boutet de Monvel, Anne; Kostenko, Aleksey; Shepelsky, Dmitry; Teschl, Gerald, Long-Time Asymptotics for the Camassa–Holm Equation, SIAM J. Math. Anal. 41 (4), 2009, 41 (4): 1559–1588, arXiv:0902.0391 , doi:10.1137/090748500 
• Bressan, Alberto; Constantin, Adrian, Global conservative solutions of the Camassa–Holm equation, Arch. Ration. Mech. Anal. 183 (2), 2007a, 183 (2): 215–239 [2013-12-30], Bibcode:2007ArRMA.183..215B, doi:10.1007/s00205-006-0010-z, （原始内容存档于2020-08-04） 
• Bressan, Alberto; Constantin, Adrian, Global dissipative solutions of the Camassa–Holm equation, Anal. Appl. 5, 2007b, 5: 1–27 [2013-12-30], doi:10.1142/S0219530507000857, （原始内容存档于2016-03-05） 
• Camassa, Roberto; Holm, Darryl D., An integrable shallow water equation with peaked solitons, Phys. Rev. Lett. 71 (11), 1993, 71 (11): 1661–1664, Bibcode:1993PhRvL..71.1661C, arXiv:patt-sol/9305002 , doi:10.1103/PhysRevLett.71.1661 
• Constantin, Adrian, Existence of permanent and breaking waves for a shallow water equation: a geometric approach, Annales de l'Institut Fourier 50 (2), 2000, 50 (2): 321–362 [2013-12-30], （原始内容存档于2016-03-03） 
• Constantin, Adrian, On the scattering problem for the Camassa–Holm equation, R. Soc. Lond. Proc. Ser. A Math. Phys. Eng. Sci. 457 (2008), 2001, 457 (2008): 953–970, Bibcode:2001RSPSA.457..953C, doi:10.1098/rspa.2000.0701 
• Constantin, Adrian; Escher, Joachim, Wave breaking for nonlinear nonlocal shallow water equations, Acta Math. 181 (2), 1998b, 181 (2): 229–243, doi:10.1007/BF02392586 
• Constantin, Adrian; Escher, Joachim, On the blow-up rate and the blow-up set of breaking waves for a shallow water equation, Math. Z. 233 (1), 2000, 233 (1): 75–91, doi:10.1007/PL00004793 
• Constantin, Adrian; McKean, Henry P., A shallow water equation on the circle, Commun. Pure Appl. Math. 52 (8), 1999, 52 (8): 949–982, doi:10.1002/(SICI)1097-0312(199908)52:8<949::AID-CPA3>3.0.CO;2-D 
• Constantin, Adrian; Strauss, Walter A., Stability of peakons, Comm. Pure Appl. Math., 2000, 53 (5): 603–610, doi:10.1002/(SICI)1097-0312(200005)53:5<603::AID-CPA3>3.0.CO;2-L 
• Constantin, Adrian; Strauss, Walter A., Stability of the Camassa–Holm solitons, J. Nonlinear Sci., 2002, 12 (4): 415–422, Bibcode:2002JNS....12..415C, doi:10.1007/s00332-002-0517-x 
• Constantin, Adrian; Gerdjikov, Vladimir S.; Ivanov, Rossen I., Inverse scattering transform for the Camassa–Holm equation, Inverse Problems 22 (6), 2006, 22 (6): 2197–2207, Bibcode:2006InvPr..22.2197C, arXiv:nlin/0603019 , doi:10.1088/0266-5611/22/6/017 
• Eckhardt, Jonathan; Teschl, Gerald, On the isospectral problem of the dispersionless Camassa-Holm equation, Adv. Math. 235 (1), 2013, 235 (1): 469–495, arXiv:1205.5831 , doi:10.1016/j.aim.2012.12.006 
• Loubet, Enrique, About the explicit characterization of Hamiltonians of the Camassa–Holm hierarchy, J. Nonlinear Math. Phys. 12 (1), 2005, 12 (1): 135–143, Bibcode:2005JNMP...12..135L, doi:10.2991/jnmp.2005.12.1.11 
• McKean, Henry P., Fredholm determinants and the Camassa–Holm hierarchy, Comm. Pure Appl. Math. 56 (5), 2003a, 56 (5): 638–680, doi:10.1002/cpa.10069 
• McKean, Henry P., Breakdown of the Camassa–Holm equation, Comm. Pure Appl. Math. 57 (3), 2004, 57 (3): 416–418, doi:10.1002/cpa.20003 
• Parker, Allen, On the Camassa–Holm equation and a direct method of solution. III. N-soliton solutions, Proc. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 461 (2064), 2005b, 461 (2064): 3893–3911, Bibcode:2005RSPSA.461.3893P, doi:10.1098/rspa.2005.1537 
• Liao, S.J., Do peaked solitary water waves indeed exist?, Communications in Nonlinear Science and Numerical Simulation, 2013, doi:10.1016/j.cnsns.2013.09.042 

1. *谷超豪 《孤立子理论中的达布变换及其几何应用》 上海科学技术出版社
2. *阎振亚著 《复杂非线性波的构造性理论及其应用》 科学出版社 2007年
3. 李志斌编著 《非线性数学物理方程的行波解》 科学出版社
4. 王东明著 《消去法及其应用》 科学出版社 2002
5. *何青 王丽芬编著 《Maple 教程》 科学出版社 2010 ISBN 9787030177445
6. Graham W. Griffiths William E.Shiesser Traveling Wave Analysis of Partial Differential p135 Equations Academy Press
7. Richard H. Enns George C. McCGuire, Nonlinear Physics Birkhauser,1997
8. Inna Shingareva, Carlos Lizárraga-Celaya,Solving Nonlinear Partial Differential Equations with Maple Springer.
9. Eryk Infeld and George Rowlands,Nonlinear Waves,Solitons and Chaos,Cambridge 2000
10. Saber Elaydi,An Introduction to Difference Equationns, Springer 2000
11. Dongming Wang, Elimination Practice,Imperial College Press 2004
12. David Betounes, Partial Differential Equations for Computational Science: With Maple and Vector Analysis Springer, 1998 ISBN 9780387983004
13. George Articolo Partial Differential Equations & Boundary Value Problems with Maple V Academic Press 1998 ISBN 9780120644759