五階六邊形鑲嵌
 龐加萊圓盤模型 | ||
| 類別 | 雙曲正鑲嵌 | |
|---|---|---|
| 對偶多面體 | 六階五邊形鑲嵌 | |
| 數學表示法 | ||
| 考克斯特符號 | ||
| 施萊夫利符號 | {6,5} | |
| 威佐夫符號 | 5 | 6 2 | |
| 組成與佈局 | ||
| 頂點圖 | 65 | |
| 對稱性 | ||
| 對稱群 | [6,5], (*652) | |
| 旋轉對稱群 | [6,5]+, (652) | |
| 圖像 | ||
| ||
在幾何學中,五階六邊形鑲嵌(英語:Order-5 hexagonal tiling)是由六邊形組成的雙曲面正鑲嵌圖,在施萊夫利符號中用{6,5}表示。五階六邊形鑲嵌即每個頂點皆為五個六邊形的公共頂點,頂點周圍包含了五個不重疊的六邊形,因此無法因此無法在平面作出,但可以在雙曲面上作出[1],或以正則地區圖的形式存在。
性質
[編輯]五階六邊形鑲嵌是指每個頂點皆為五個六邊形的公共頂點所構成的鑲嵌結構。一般而言這種鑲嵌結構僅能存在於雙曲面上並無窮延伸[2]。然而透過取出區面的局部將其轉成其他虧格的環面則可將其轉換為有限的正則地區圖[3]。在施萊夫利符號中五階六邊形鑲嵌可以用{6,5}表示,這個符號表是每個頂點皆為五個六邊形的公共頂點。而在正則地區圖中,五階六邊形鑲嵌會表達為{6,5}p,其中下標的p表示這個正則地區圖對應的皮特里多邊形為p邊形[4]。
正則地區圖
[編輯]做為有限的正則地區圖,五階六邊形鑲嵌從虧格為9開始存在[5],其中可定向的{6,5}正則地區圖有皮特里多邊形為六邊形、八邊形、十邊形、十二邊形等的正則地區圖[6],不可定向的有皮特里多邊形為四邊形、五邊形、六邊形和十邊形的正則地區圖[7]。
| 可定向性 | 虧格 | 施萊夫利符號 | 對應多面體 | 面數 | 邊數 | 頂點數 |
|---|---|---|---|---|---|---|
| 可定向[6] | 1 | {6,5} | 雙曲無限面體 (雙曲正鑲嵌) |
|||
| 9 | {6,5}4 | 二十面體 | 20 | 60 | 24 | |
| {6,5}10 | 二十面體 | 20 | 60 | 24 | ||
| 17 | {6,5}8 | 四十面體 | 40 | 120 | 48 | |
| 41 | {6,5}10 | 一百面體 | 100 | 300 | 120 | |
| {6,5}20 | 一百面體 | 100 | 300 | 120 | ||
| 45 | {6,5}6 | 一百一十面體 | 110 | 330 | 132 | |
| {6,5}12 | 一百一十面體 | 110 | 330 | 132 | ||
| 49 | {6,5}6 | 一百二十面體 | 120 | 360 | 144 | |
| 55 | {6,5}10 | 一百三十五面體 | 135 | 405 | 162 | |
| 65 | {6,5}10 | 一百六十面體 | 160 | 480 | 192 | |
| 81 | {6,5}40 | 二百面體 | 200 | 600 | 240 | |
| 89 | {6,5}6 | 二百二十面體 | 220 | 660 | 264 | |
| {6,5}12 | 二百二十面體 | 220 | 660 | 264 | ||
| 不可定向[7] | 10 | {6,5}4 | 十面體 | 10 | 30 | 12 |
| {6,5}5 | 十面體 | 10 | 30 | 12 | ||
| {6,5}10 | 十面體 | 10 | 30 | 12 | ||
| 42 | {6,5}5 | 五十面體 | 50 | 150 | 60 | |
| 46 | {6,5}6 | 五十五面體 | 55 | 165 | 66 | |
| 50 | {6,5}6 | 六十面體 | 60 | 180 | 72 | |
| 50 | {6,5}6 | 六十面體 | 60 | 180 | 72 | |
| 66 | {6,5}6 | 八十面體 | 80 | 240 | 96 | |
| 90 | {6,5}6 | 一百一十面體 | 110 | 330 | 132 |
相關多面體與鑲嵌
[編輯]相關多面體
[編輯]皮特里大十二面體
[編輯] 以不同顏色表示每個面 | |
| 類別 | 皮特里對偶 正則地區圖 |
|---|---|
| 數學表示法 | |
| 施萊夫利符號 | {5,5/2}π |
| 性質 | |
| 面 | 10 |
| 邊 | 30 |
| 頂點 | 12 |
| 歐拉特徵數 | F=10, E=30, V=12 (χ=-8) |
| 二面角 | (不存在) |
| 對稱性 | |
| 對稱群 | Ih, H3, [5,3], *532 |
| 特性 | |
| 扭歪、正則 | |
皮特里大十二面體是大十二面體的皮特里對偶,可以透過將原有大十二面體上取皮特里多邊形構成,換句話說,皮特里大十二面體為由大十二面體的皮特里多邊形構成的立體[8][9]。由於大十二面體的皮特里多邊形為扭歪六邊形,因此無法確立其封閉範圍,故無法計算其表面積和體積。
 大十二面體 |
 皮特里大十二面體 |
由於大十二面體與小星形十二面體對應相同的正則地區圖[10],因此皮特里大十二面體對應的正則地區圖也與皮特里小星形十二面體相同,皆由10個面、30條邊和12個頂點組成[11]。
皮特里大十二面體是一個不可定向且虧格為10的幾何結構[10]。皮特里大十二面體由10個扭歪六邊形組成,每個頂點都是5個扭歪六邊形的公共頂點,其對應的正則地區圖為五階六邊形鑲嵌,並具有五邊形的皮特里多邊形,在施萊夫利符號中可以用{6,5}5表示[10]。
 大十二面體的皮特里多邊形 |
 構成皮特里大十二面體的扭歪六邊形面 |
皮特里小星形十二面體
[編輯] 以不同顏色表示每個面 | |
| 類別 | 皮特里對偶 正則地區圖 |
|---|---|
| 數學表示法 | |
| 施萊夫利符號 | {5/2,5}π |
| 性質 | |
| 面 | 10 |
| 邊 | 30 |
| 頂點 | 12 |
| 歐拉特徵數 | F=10, E=30, V=12 (χ=-8) |
| 二面角 | (不存在) |
| 對稱性 | |
| 對稱群 | Ih, H3, [5,3], *532 |
| 特性 | |
| 扭歪、正則 | |
皮特里小星形十二面體是小星形十二面體的皮特里對偶,可以透過將原有小星形十二面體上取皮特里多邊形構成,其拓樸結構與皮特里大十二面體同構[12]。是施萊夫利符號為{6,5}的120階元素對稱性抽象多面體的一種具象化結果[13]。 皮特里小星形十二面體、小星形十二面體、大十二面體、皮特里大十二面體的關係如下:[12]
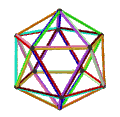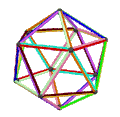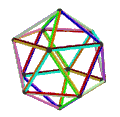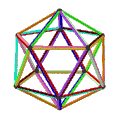 皮特里大十二面體 |
 大十二面體 |
 小星形十二面體 |
 皮特里小星形十二面體 |
 小星形十二面體 |
 皮特里小星形十二面體 |
大十二面體與小星形十二面體對應相同的正則地區圖[10],皆由10個面、30條邊和12個頂點組成[11],且每個頂點都是5個扭歪六邊形的公共頂點,對應的正則地區圖同為五階六邊形鑲嵌,並具有五邊形的皮特里多邊形,在施萊夫利符號中可以用{6,5}5表示[10]。然而其構成面面有些許不同,且來自不同原像,因此可以被視為{6,5}的不同具象化方式[13]。
 小星形十二面體的皮特里多邊形 |
 小星形十二面體的皮特里多邊形 正交投影 |
 構成皮特里小星形十二面體 的扭歪六邊形面 |
相關鑲嵌圖
[編輯]該鑲嵌在拓樸學中也和每個頂點為五個多邊形的公共頂點的多面體及鑲嵌相關,這類幾何結構施萊夫利符號皆為{n,5}、考克斯特符號為![]()
![]()
![]()
![]()
![]() ,其中n從2到無窮。[14]
,其中n從2到無窮。[14]
| 球面鑲嵌 | 雙曲面鑲嵌 | |||||||
|---|---|---|---|---|---|---|---|---|
 {2,5} |
 {3,5} |
 {4,5} |
 {5,5} |
 {6,5} |
 {7,5} |
 {8,5} |
... |  {∞,5} |
該鑲嵌在拓樸學上頂點圖為65,其餘頂點圖為6n的幾何結構有:[14]
| 球面 | 歐氏 | 雙曲鑲嵌 | ||||||
|---|---|---|---|---|---|---|---|---|
 {6,2} |
 {6,3} |
 {6,4} |
 {6,5} |
 {6,6} |
 {6,7} |
 {6,8} |
... |  {6,∞} |
五階六邊形鑲嵌可以透過康威多面體變換成一系列鑲嵌圖:
| 正六邊形/五邊形鑲嵌 | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 對稱性:[6,5], (*652) | [6,5]+, (652) | [6,5+], (5*3) | [1+,6,5], (*553) | ||||||||

|

|

|

|

|

|

|

|

| |||
| {6,5} | t{6,5} | r{6,5} | 2t{6,5}=t{5,6} | 2r{6,5}={5,6} | rr{6,5} | tr{6,5} | sr{6,5} | s{5,6} | h{6,5} | ||
| 對偶鑲嵌 | |||||||||||

|

|

|

|

|

|

|
|||||
| V65 | V5.12.12 | V5.6.5.6 | V6.10.10 | V56 | V4.5.4.6 | V4.10.12 | V3.3.5.3.6 | V3.3.3.5.3.5 | V(3.5)5 | ||
| [(5,5,3)] 反射對稱性均勻鑲嵌 | ||||||
|---|---|---|---|---|---|---|

|

|

|

|

|

|

|
參見
[編輯]參考資料
[編輯]- ^ John H. Conway; Heidi Burgiel; Chaim Goodman-Strass. The Symmetries of Things. A K Peters. [2022-05-28]. ISBN 978-1-56881-220-5. (原始內容存檔於2010-09-19).(Chapter 19, The Hyperbolic Archimedean Tessellations)
- ^ Chapter 10: Regular honeycombs in hyperbolic space. The Beauty of Geometry: Twelve Essays. Dover Publications. 1999. ISBN 0-486-40919-8. LCCN 99035678.
- ^ Razafindrazaka, Faniry and Polthier, Konrad; et al. Regular surfaces and regular maps. Proceedings of Bridges. 2014: 225–234.
- ^ Schlafli, Glossary. weddslist.com. [2021-08-09]. (原始內容存檔於2021-05-07).
- ^ Regular maps in the orientable surface of genus 9. weddslist.com. [2021-08-09]. (原始內容存檔於2021-10-19).
- ^ 6.0 6.1 Regular maps in the orientable surface of genus 17 (頁面存檔備份,存於網際網路檔案館), genus 41 (頁面存檔備份,存於網際網路檔案館), genus 45 (頁面存檔備份,存於網際網路檔案館), genus 49 (頁面存檔備份,存於網際網路檔案館), genus 55 (頁面存檔備份,存於網際網路檔案館), genus 65 (頁面存檔備份,存於網際網路檔案館), genus 81 (頁面存檔備份,存於網際網路檔案館), genus 89. weddslist.com. [2021-08-09]. (原始內容存檔於2021-08-09).
- ^ 7.0 7.1 Regular maps in the non-orientable surface of genus 10 (頁面存檔備份,存於網際網路檔案館), genus 42 (頁面存檔備份,存於網際網路檔案館), genus 46 (頁面存檔備份,存於網際網路檔案館), genus 50 (頁面存檔備份,存於網際網路檔案館), genus 66 (頁面存檔備份,存於網際網路檔案館), genus 90. weddslist.com. [2021-08-09]. (原始內容存檔於2021-08-09).
- ^ Gorini, Catherine A., Geometry at Work, MAA Notes 53, Cambridge University Press: 181, 2000, ISBN 9780883851647
- ^ McMullen, Peter. Rigidity of Regular Polytopes. Rigidity and Symmetry (Springer). 2014: 253––278.
- ^ 10.0 10.1 10.2 10.3 10.4 S4:{5,5}. Regular Map database - map details, weddslist.com. [2021-07-30].
- ^ 11.0 11.1 N10.5′. Regular Map database - map details, weddslist.com. [2021-07-30]. (原始內容存檔於2021-08-05).
- ^ 12.0 12.1 McMullen, P., Schulte, E. Regular Polytopes in Ordinary Space. Discrete & Computational Geometry. 1997/06/01, 17 (4): pp.449-478 [2021-08-09]. ISSN 1432-0444. doi:10.1007/PL00009304. (原始內容存檔於2018-06-03).
- ^ 13.0 13.1 Aranas, Jonn Angel L and Loyola, Mark L. Geometric realizations of abstract regular polyhedra with automorphism group H3. Acta Crystallographica Section A: Foundations and Advances (International Union of Crystallography). 2020, 76 (3): 358–368.
- ^ 14.0 14.1 Klitzing, Richard. Noble Polytopes. bendwavy.org. [2021-08-09]. (原始內容存檔於2021-10-24).
外部連結
[編輯]- 埃里克·韋斯坦因. Hyperbolic tiling. MathWorld.
- 埃里克·韋斯坦因. Poincaré hyperbolic disk. MathWorld.
- Hyperbolic and Spherical Tiling Gallery(頁面存檔備份,存於網際網路檔案館)
- KaleidoTile 3: Educational software to create spherical, planar and hyperbolic tilings (頁面存檔備份,存於網際網路檔案館)
- Hyperbolic Planar Tessellations, Don Hatch(頁面存檔備份,存於網際網路檔案館)



![{\displaystyle {\ce {<->[\pi]}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8deea10ebe9c2b65f039af2e77ae19399fa9a1ee)

![{\displaystyle {\ce {<->[\delta]}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/59bb43307cba9af023529bb69e0c25987ad28eab)


